(Solved): You may need to use the appropriate appendix table or technology to answer this question. Fourteen ...

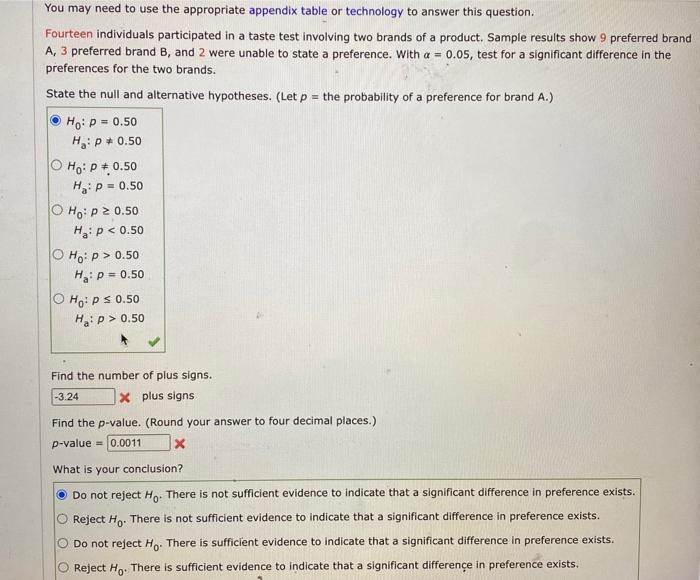
You may need to use the appropriate appendix table or technology to answer this question. Fourteen individuals participated in a taste test involving two brands of a product. Sample results show 9 preferred brand \( A \), 3 preferred brand \( B \), and 2 were unable to state a preference. With \( \alpha=0.05 \), test for a significant difference in the preferences for the two brands. State the null and alternative hypotheses. (Let \( p= \) the probability of a preference for brand A.) \( H_{0}: p=0.50 \) \( H_{a}: p \neq 0.50 \) \( H_{0}: p \neq 0.50 \) \( H_{a}: p=0.50 \) \( H_{0}: p \geq 0.50 \) \( H_{a}: p<0.50 \) \( H_{0}: p>0.50 \) \( H_{a}: p=0.50 \) \( H_{0}: p \leq 0.50 \) \( H_{a}: p>0.50 \) Find the number of plus signs. X plus signs Find the \( p \)-value. (Round your answer to four decimal places.) \( p \)-value \( = \) What is your conclusion? Do not reject \( H_{0} \). There is not sufficient evidence to indicate that a significant difference in preference exists. Reject \( H_{0} \). There is not sufficient evidence to indicate that a significant difference in preference exists. Do not reject \( H_{0} \). There is sufficient evidence to indicate that a significant difference in preference exists. Reject \( H_{0} \). There is sufficient evidence to indicate that a significant differençe in preference exists.