(Solved): Write the sum using summation notation. There may be multiple representations. Use \( i \) as the i ...

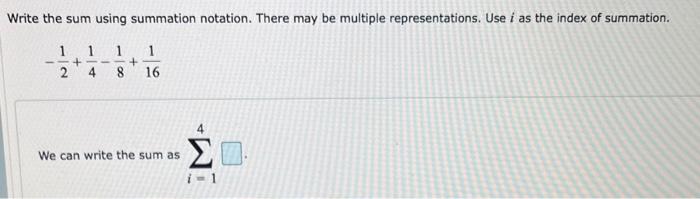
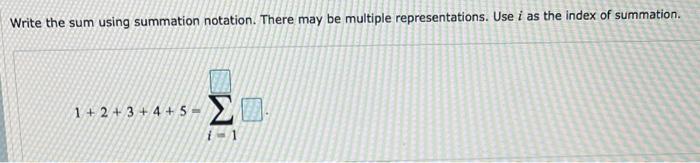
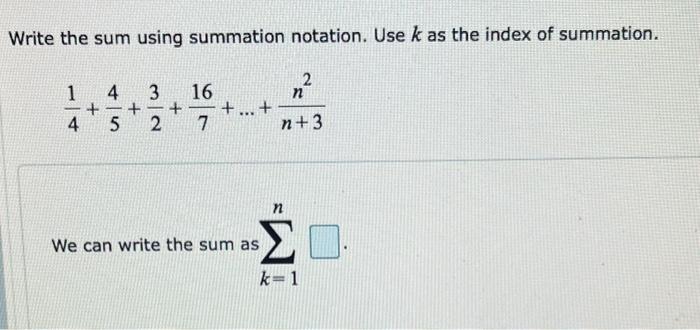
Write the sum using summation notation. There may be multiple representations. Use \( i \) as the index of summation. \[ -\frac{1}{2}+\frac{1}{4}-\frac{1}{8}+\frac{1}{16} \] We can write the sum as Write the sum using summation notation. There may be multiple representations. Use \( i \) as the index of summation. \[ 1+2+3+4+5=\sum_{i=1} \] Write the sum using summation notation. Use \( k \) as the index of summation. \[ \frac{1}{4}+\frac{4}{5}+\frac{3}{2}+\frac{16}{7}+\ldots+\frac{n^{2}}{n+3} \] We can write the sum as \( \sum_{k=1}^{n} \) (a) Evaluate \( \sum_{i=1}^{n}(-1)^{i+3} \) if \( n \) is even. \[ \sum_{i=1}^{n}(-1)^{i+3}= \] Part 2 of 2 (b) Evaluate \( \sum_{i=1}^{n}(-1)^{i+3} \) if \( n \) is odd. \[ \sum_{i=1}^{n}(-1)^{i+3}= \] Question 18 of 22 ( 1 point) \( \mid \) Attempt 1 of Unlimited Find the sum. \[ \sum_{i=1}^{4}(i+1)(i+3)= \] Find the sum. Express the sum as a fraction in lowest terms. \[ \sum_{j=2}^{6}\left(\frac{1}{3}\right)^{j}= \] Find the sum. \[ \sum_{i=1}^{4}(5 i-7)= \]