(Solved): While often the eigenvalues for the second order linear systems end up being negative in applicati ...

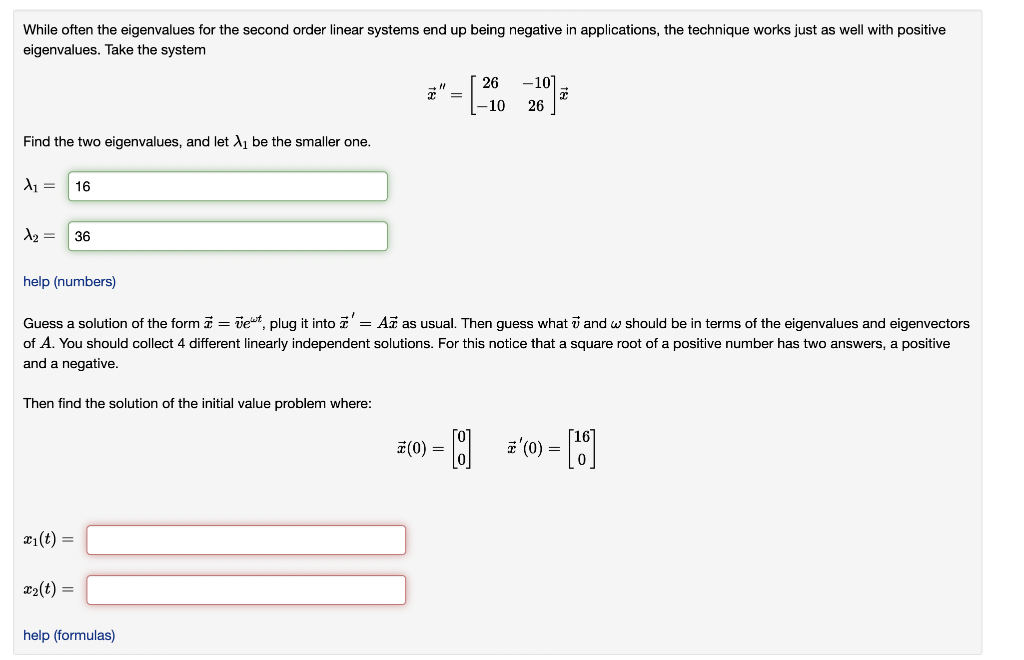
While often the eigenvalues for the second order linear systems end up being negative in applications, the technique works just as well with positive eigenvalues. Take the system ${\vec{x}}^{??}=\left[\begin{array}{cc}26& ?10\\ ?10& 26\end{array}\right]\vec{x}$ Find the two eigenvalues, and let ${?}_1$ be the smaller one. ${?}_1=$ ${?}_2=$ help (numbers) Guess a solution of the form $\vec{x}=\vec{v}{e}^{?t}$, plug it into ${\vec{x}}^{?}=A\vec{x}$ as usual. Then guess what $\vec{v}$ and $?$ should be in terms of the eigenvalues and eigenvectors of $A$. You should collect 4 different linearly independent solutions. For this notice that a square root of a positive number has two answers, a positive and a negative. Then find the solution of the initial value problem where: $\vec{x}(0)=\left[\begin{array}{l}0\\ 0\end{array}\right]{\vec{x}}^{?}(0)=\left[\begin{array}{c}16\\ 0\end{array}\right]$ $x_1(t)=$ $x_2(t)=$