(Solved): We modeled a rotational door system during the class (Figure 4). The rotational angle \( \theta(t) ...

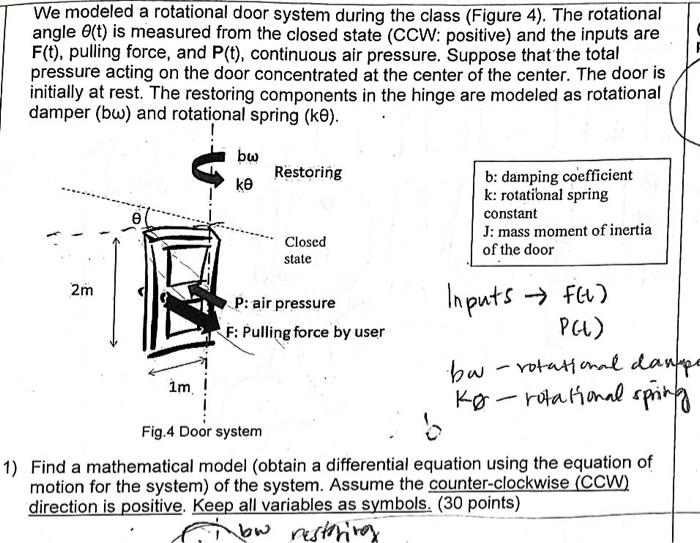
We modeled a rotational door system during the class (Figure 4). The rotational angle \( \theta(t) \) is measured from the closed state (CCW: positive) and the inputs are \( F(t) \), pulling force, and \( P(t) \), continuous air pressure. Suppose that the total pressure acting on the door concentrated at the center of the center. The door is initially at rest. The restoring components in the hinge are modeled as rotational damper \( (\mathrm{b} \omega) \) and rotational sbrina \( (\mathrm{k} \theta) \). \begin{tabular}{l} \hline b: damping coefficient \\ k: rotational spring \\ constant \\ J: mass moment of inertia \\ of the door \\ \hline \end{tabular} \( \begin{aligned} \text { Inputs } \rightarrow & f(t) \\ & P(t)\end{aligned} \) bw - rotational day Kor - rotational spring Find a mathematical model (obtain a differential equation using the equation of motion for the system) of the system. Assume the counter-clockwise (CCW) direction is positive. Keep all variables as symbols. (30 points)