(Solved): Water is flowing in a trapezoidal channel at a rate of \( Q=20 \mathrm{~m}^{3} / \mathrm{s} \). Th ...

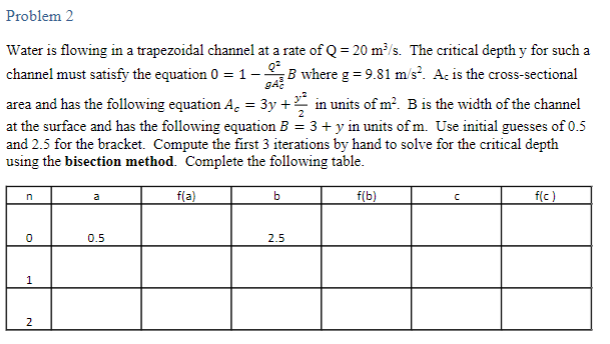
Water is flowing in a trapezoidal channel at a rate of \( Q=20 \mathrm{~m}^{3} / \mathrm{s} \). The critical depth y for such a channel must satisfy the equation \( 0=1-\frac{Q^{2}}{g A_{c}^{3}} B \) where \( g=9.81 \mathrm{~m} / \mathrm{s}^{2} . A_{c} \) is the cross-sectional area and has the following equation \( A_{c}=3 y+\frac{y^{2}}{2} \) in units of \( \mathrm{m}^{2} \). B is the width of the channel at the surface and has the following equation \( B=3+y \) in units of \( \mathrm{m} \). Use initial guesses of \( 0.5 \) and \( 2.5 \) for the bracket. Compute the first 3 iterations by hand to solve for the critical depth using the bisection method. Complete the following table.