(Solved): Use the method for solving homogeneous equations to solve the following differential equation. \[ ...

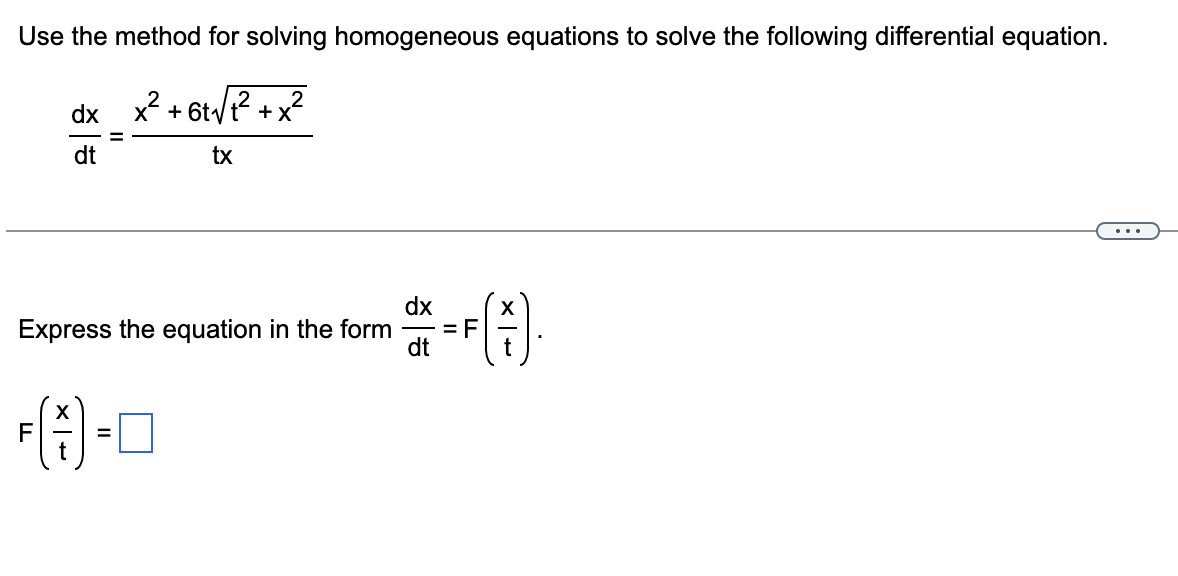
Use the method for solving homogeneous equations to solve the following differential equation. \[ \frac{d x}{d t}=\frac{x^{2}+6 t \sqrt{t^{2}+x^{2}}}{t x} \] Express the equation in the form \( \frac{d x}{d t}=F\left(\frac{x}{t}\right) \). \[ F\left(\frac{x}{t}\right)= \] \[ (2 x-y) d x+(4 x+y-3) d y=0 \] \[ \begin{array}{r} 2 h-k=x \\ 4 h+k=y \\ 2 h-k=0 \\ 4 h+k=3 \\ \text { D. } \quad-2 h-k=0 \\ -4 h+k=3 \end{array} \] Find the differential equation \( \frac{d v}{d u} \) in terms of \( v \) and \( u \). \[ \frac{d v}{d u}=\frac{v-2 u}{4 u+v} \] Let \( z=\frac{v}{u} \). Find the differential equation \( \frac{d z}{d u} \). 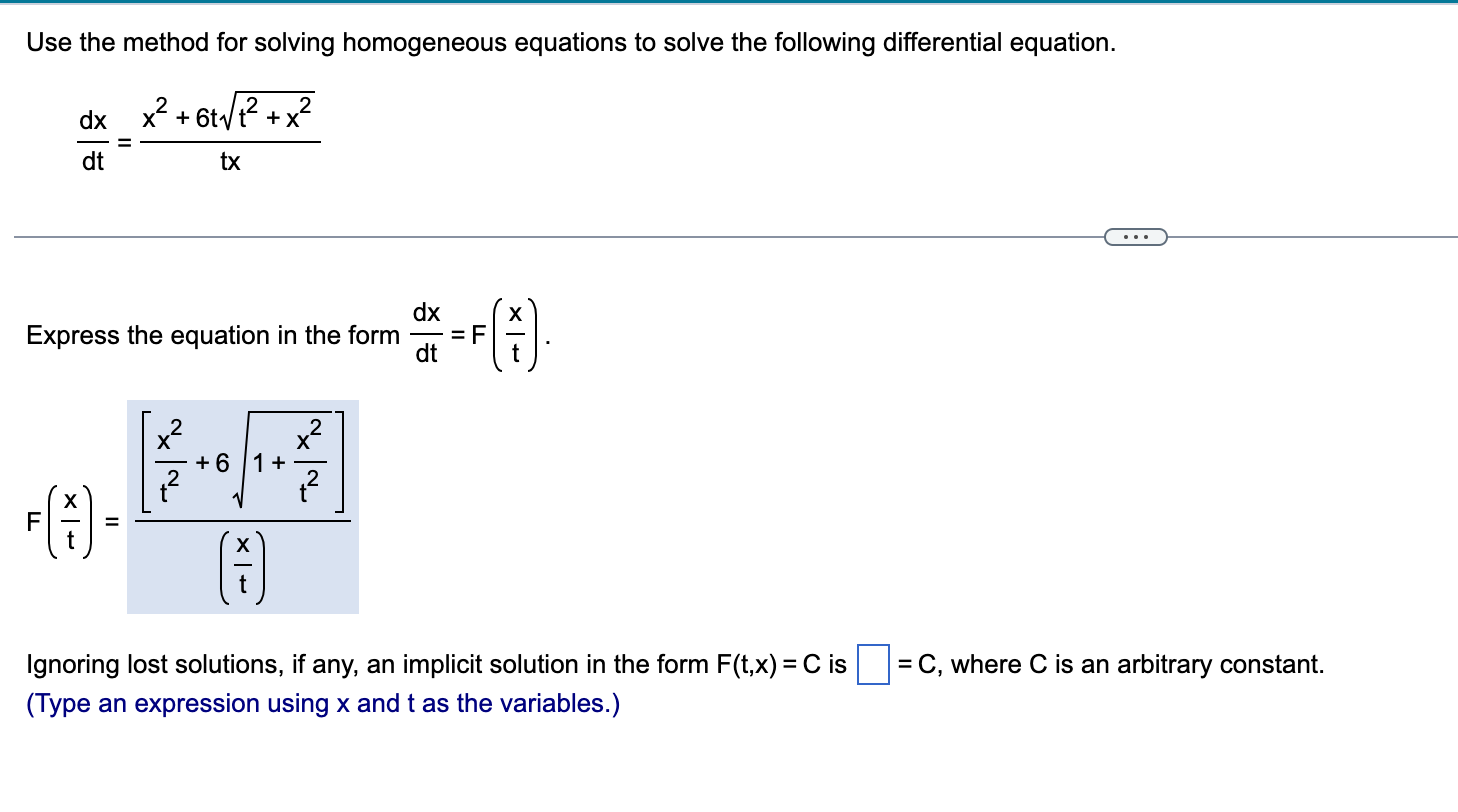\( \frac{d z}{d u}= \) Use the method for solving homogeneous equations to solve the following differential equation. \[ \frac{d x}{d t}=\frac{x^{2}+6 t \sqrt{t^{2}+x^{2}}}{t x} \] Express the equation in the form \( \frac{d x}{d t}=F\left(\frac{x}{t}\right) \). \[ F\left(\frac{x}{t}\right)=\frac{\left[\frac{x^{2}}{t^{2}}+6 \sqrt{1+\frac{x^{2}}{t^{2}}}\right]}{\left(\frac{x}{t}\right)} \] Ignoring lost solutions, if any, an implicit solution in the form \( \mathrm{F}(\mathrm{t}, \mathrm{x})=\mathrm{C} \) is \( \quad=\mathrm{C} \), where \( \mathrm{C} \) is an arbitrary constant. (Type an expression using \( x \) and \( t \) as the variables.) \[ \frac{d y}{d x}+\frac{y}{x}=5 x^{6} y^{2} \] Ignoring lost solutions, if any, the general solution is \( y= \) (Type an expression using \( x \) as the variable. Do not use \( d, D, e, E, i \), or I as arbitrary constants since these letters already have defined meanings.)