(Solved): Use the first derivative test to locate the relative extrema of the function in the given domain, ...

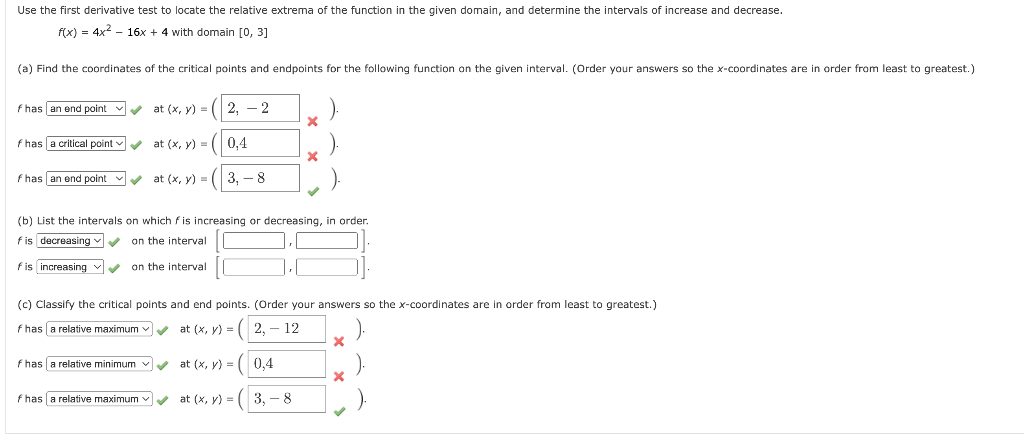
Use the first derivative test to locate the relative extrema of the function in the given domain, and determine the intervals of increase and decrease. \[ f(x)=4 x^{2}-16 x+4 \text { with domain }[0,3] \] \( \begin{array}{ll}f \text { has } & \text { at }(x, y)=( \\ f \text { has } & \text { at }(x, y)=( \\ f \text { has } & \text { at }(x, y)=(\end{array} \) (b) List the intervals on which \( f \) is increasing or decreasing, in order. \( f \) is on the interval \( [ \), \( f \) is (c) Classify the critical points and end points. (Order your answers so the \( x \)-coordinates are in order from least to greatest.) fhas has fhas at \( (x, y)=\left(\begin{array}{c}\infty\end{array}\right) \). at \( (x, y)=\left(\begin{array}{l}x\end{array}\right) \). at \( (x, y)=(\quad) \).