(Solved): Use a \( 0.05 \) significance level to test the clain that there is a difference between the actual ...

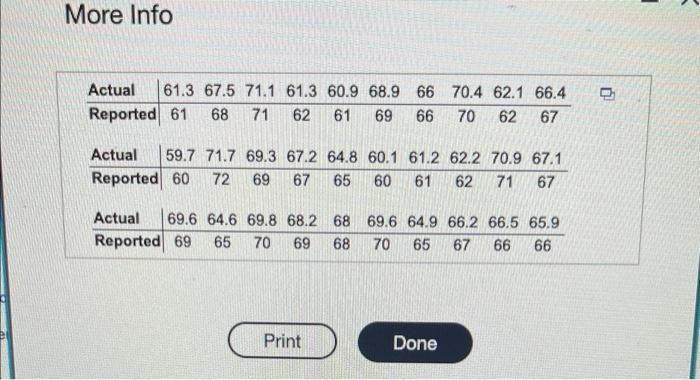
Use a \( 0.05 \) significance level to test the clain that there is a difference between the actual and teported heights, in inches, for \( 12-16 \) year old boys. The data is listed in that table below. Click the icon to view the data table of the reported heights. Let \( \mu_{1} \) denote the median of the first variable and \( \mu_{2} \) denoth the median of the second variabla. What are the null and asernative hypotheses? A. \( \mathrm{H}_{0} \mathrm{H}_{1} \geq \mathrm{H}_{2} \) B. \( M_{0}=\mu_{1}=\mu_{2} \) \( \mathrm{H}_{1}: \mu_{1}<\mu_{2} \) \( \mathrm{H}_{1}: \mu_{1}<\mu_{2} \) c. \( \mathrm{H}_{0}: \mathrm{H}_{1}+\mathrm{H}_{2} \) D. \( \mathrm{H}_{0}: \mathrm{H}_{1}=\mathrm{H}_{2} \) \( H_{1}: \mu_{1}=\mu_{2} \) \( H_{1}: \mu_{1} F \mu_{2} \). Find the test startwe. Test stanistic \( = \) (Round to two decimal places as needed) Find the Pivalue. P.yalue = (Reund to for decimal places ns needed) Is there wifteient evisence to suppor \( \mathrm{H}_{4} \) ? A. No, becavie the P.value is less than Bhe segrifcance ievel 8. Yes, becurse the Praive is greaser than the signteance levet C No. becaute the P.value is grealer than the signficance ievel, 0. Yes, becione tine Pivdue is less than the signacance level. More Info