(Solved): Two Part Question : Part A)Please Fill in the table and Find the test- statistic and p-value : Part ...

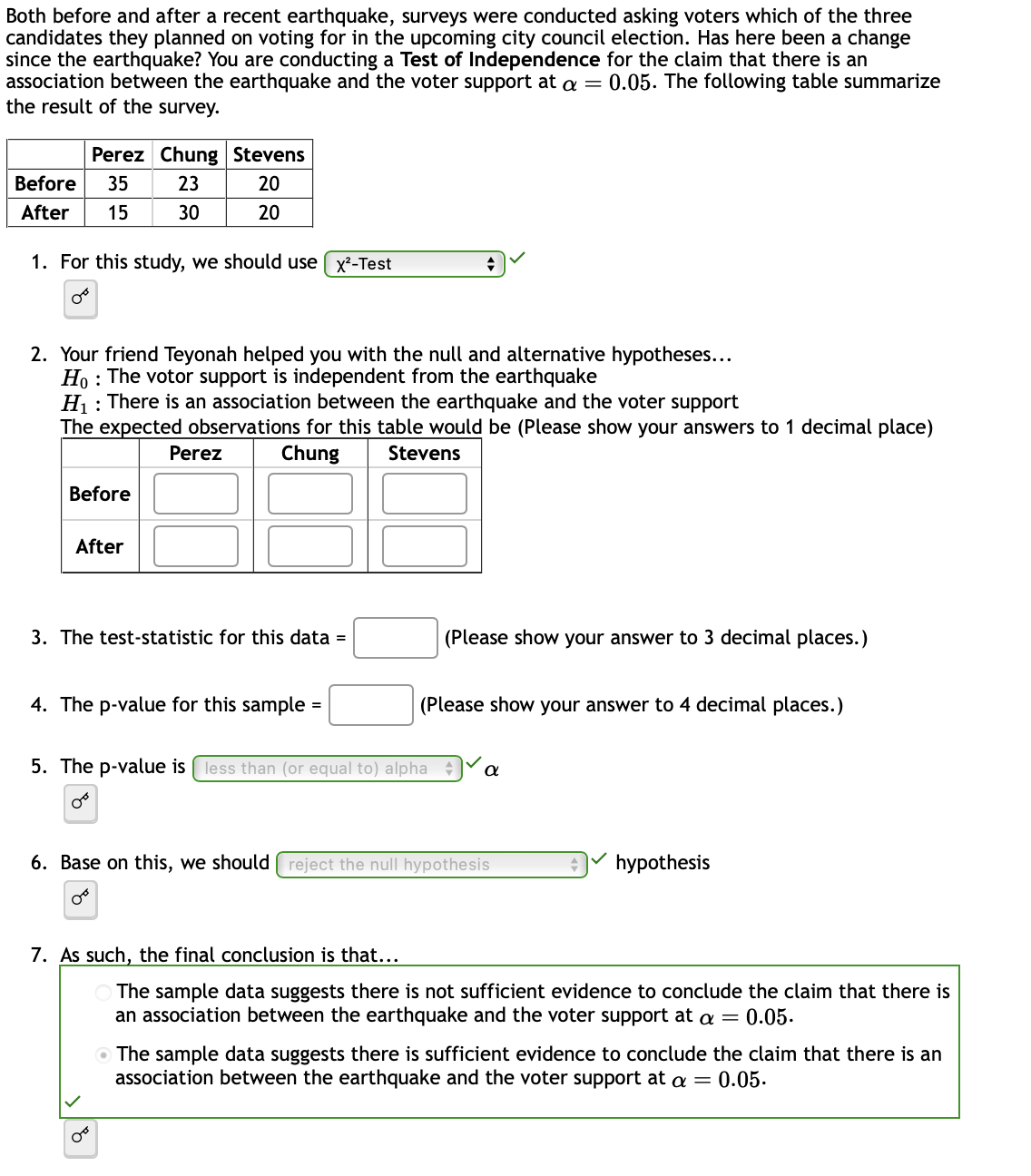
Both before and after a recent earthquake, surveys were conducted asking voters which of the three candidates they planned on voting for in the upcoming city council election. Has here been a change since the earthquake? You are conducting a Test of Independence for the claim that there is an association between the earthquake and the voter support at $?=0.05$. The following table summarize the result of the survey. 1. For this study, we should use 2. Your friend Teyonah helped you with the null and alternative hypotheses... $H_0$ : The votor support is independent from the earthquake $H_1$ : There is an association between the earthquake and the voter support The expected observations for this table would be (Please show your answers to 1 decimal place) 3. The test-statistic for this data $=$ 4. The $p$-value for this sample $=$ 5. The $p$-value is (Please show your answer to 3 decimal places.) (Please show your answer to 4 decimal places.) 6. Base on this, we should hypothesis 7. As such, the final conclusion is that... The sample data suggests there is not sufficient evidence to conclude the claim that there is an association between the earthquake and the voter support at $?=0.05$. The sample data suggests there is sufficient evidence to conclude the claim that there is an association between the earthquake and the voter support at $?=0.05$. 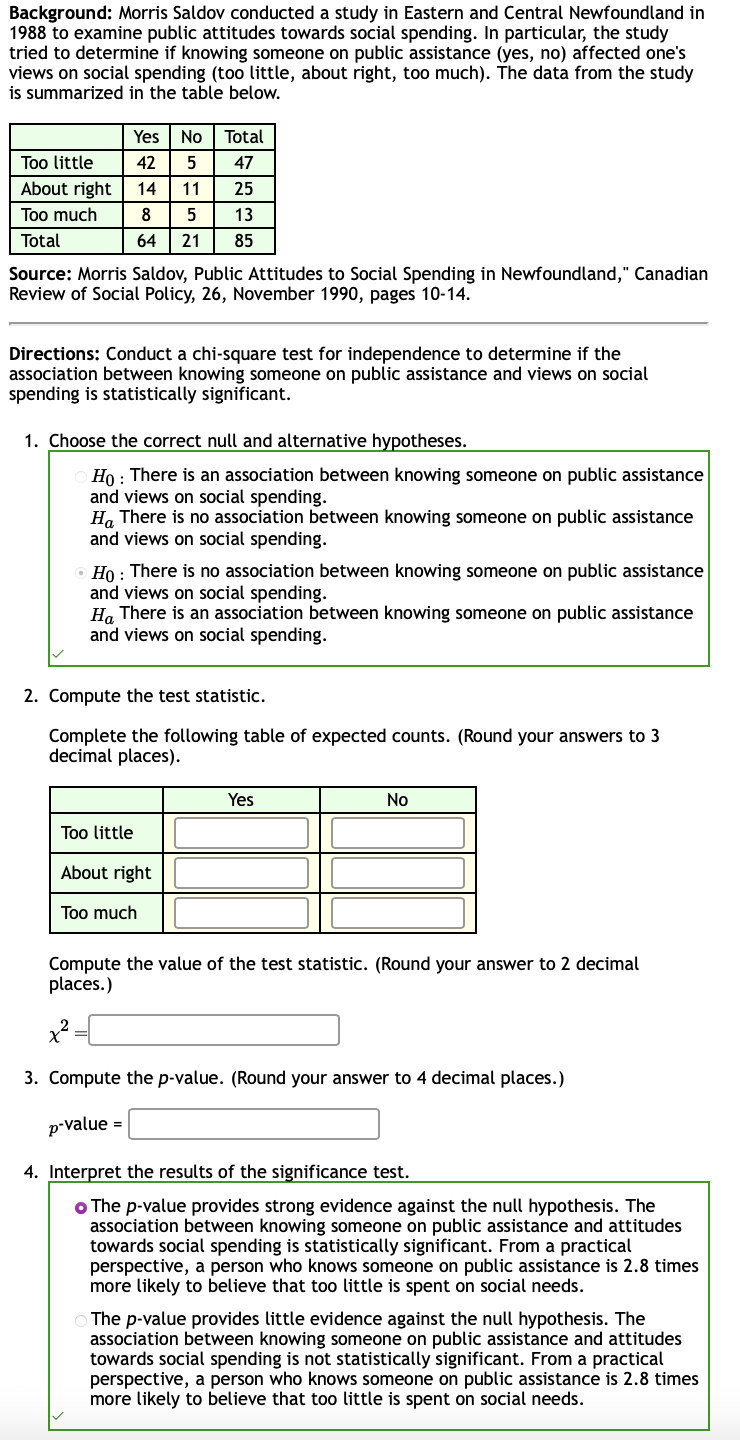Background: Morris Saldov conducted a study in Eastern and Central Newfoundland in 1988 to examine public attitudes towards social spending. In particular, the study tried to determine if knowing someone on public assistance (yes, no) affected one's views on social spending (too little, about right, too much). The data from the study is summarized in the table below. Source: Morris Saldov, Public Attitudes to Social Spending in Newfoundland," Canadian Review of Social Policy, 26, November 1990, pages 10-14. Directions: Conduct a chi-square test for independence to determine if the association between knowing someone on public assistance and views on social spending is statistically significant. 1. Choose the correct null and alternative hypotheses. $H_0$ : There is an association between knowing someone on public assistance and views on social spending. $H_a$ There is no association between knowing someone on public assistance and views on social spending. $H_0$ : There is no association between knowing someone on public assistance and views on social spending. $H_a$ There is an association between knowing someone on public assistance and views on social spending. 2. Compute the test statistic. Complete the following table of expected counts. (Round your answers to 3 decimal places). Compute the value of the test statistic. (Round your answer to 2 decimal places.) ${?}^2=$ 3. Compute the $p$-value. (Round your answer to 4 decimal places.) $p\text{-value }=$ 4. Interpret the results of the significance test. - The $p$-value provides strong evidence against the null hypothesis. The association between knowing someone on public assistance and attitudes towards social spending is statistically significant. From a practical perspective, a person who knows someone on public assistance is 2.8 times more likely to believe that too little is spent on social needs. The $p$-value provides little evidence against the null hypothesis. The association between knowing someone on public assistance and attitudes towards social spending is not statistically significant. From a practical perspective, a person who knows someone on public assistance is 2.8 times more likely to believe that too little is spent on social needs.