(Solved): To model the relationship between temperature \( (X \), in degrees) and humidity level \( (Y \), as ...

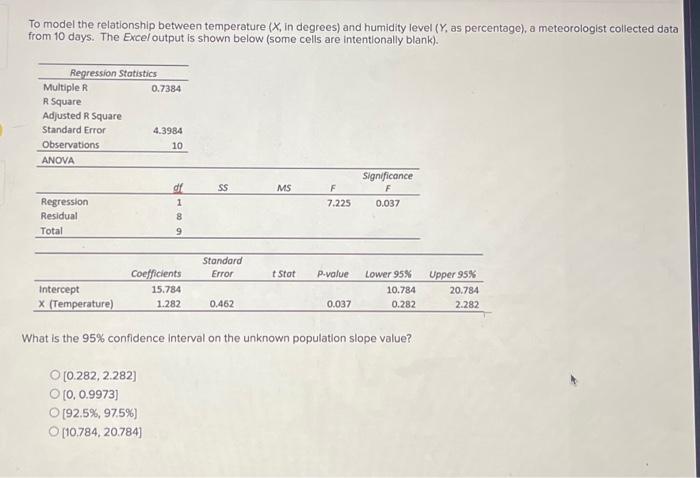
To model the relationship between temperature \( (X \), in degrees) and humidity level \( (Y \), as percentage), a meteorologist collected dati from 10 days. The Excel output is shown below (some cells are intentionally blank). What is the \( 95 \% \) confidence interval on the unknown population slope value? \[ \begin{array}{l} {[0.282,2.282]} \\ {[0.0 .9973]} \\ {[92.5 \%, 97.5 \%]} \\ {[10.784,20.784]} \end{array} \] To model the relationship between temperature \( (X \), in degrees) and humidity level ( \( Y \), as percentage), a meteorologist collected data from 10 days. The Exceloutput is shown below (some cells are intentionally blank). What percentage of the variability in humidity level is explained by its relationship with temperature? 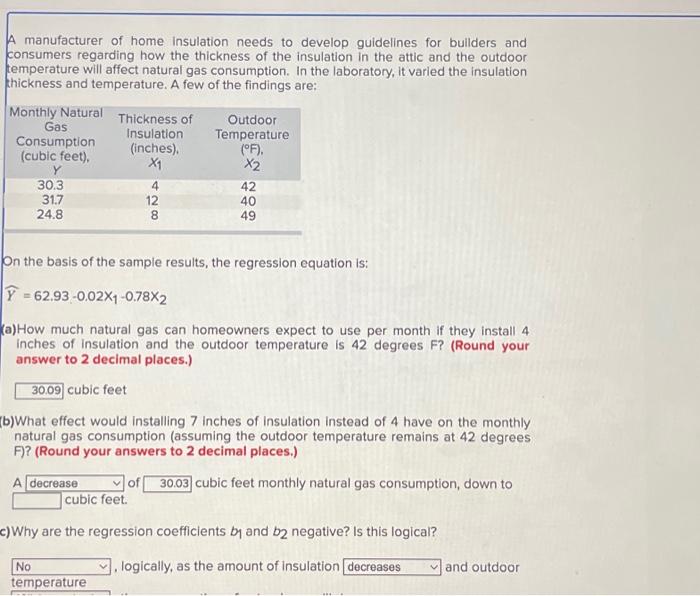\[ \begin{array}{l} 73.84 \% \\ 54.52 \% \\ 3.70 \% \\ 85.93 \% \end{array} \] A manufacturer of home insulation needs to develop guidelines for bullders and consumers regarding how the thickness of the insulation in the attic and the outdoor temperature will affect natural gas consumption. In the laboratory, it varied the insulation thickness and temperature. \( A \) few of the findings are: On the basis of the sample results, the regression equation is: \[ \widehat{Y}=62.93-0.02 \mathrm{X}_{1}-0.78 \mathrm{X}_{2} \] (a) How much natural gas can homeowners expect to use per month if they install 4 inches of insulation and the outdoor temperature is 42 degrees F? (Round your answer to 2 decimal places.) cubic feet (b)What effect would installing 7 inches of insulation instead of 4 have on the monthly natural gas consumption (assuming the outdoor temperature remains at 42 degrees F)? (Round your answers to 2 decimal places.) A of cubic feet monthly natural gas consumption, down to cubic feet. c) Why are the regression coefficients \( b_{1} \) and \( b_{2} \) negative? Is this logical? , logically, as the amount of insulation and outdoor temperature