(Solved): To calculate the mean of an exponential random variable in equation 23 , we used the fact that the ...

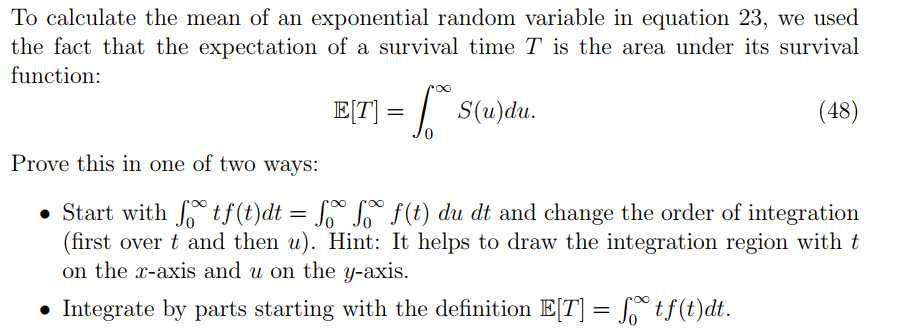
To calculate the mean of an exponential random variable in equation 23 , we used the fact that the expectation of a survival time \( T \) is the area under its survival function: \[ \mathbb{E}[T]=\int_{0}^{\infty} S(u) d u \] Prove this in one of two ways: - Start with \( \int_{0}^{\infty} t f(t) d t=\int_{0}^{\infty} \int_{0}^{\infty} f(t) d u d t \) and change the order of integration (first over \( t \) and then \( u \) ). Hint: It helps to draw the integration region with \( t \) on the \( x \)-axis and \( u \) on the \( y \)-axis. - Integrate by parts starting with the definition \( \mathbb{E}[T]=\int_{0}^{\infty} t f(t) d t \)