(Solved): to calculate dielectric response 1 - Consider a semiclassical model of the ground state of the hydr ...

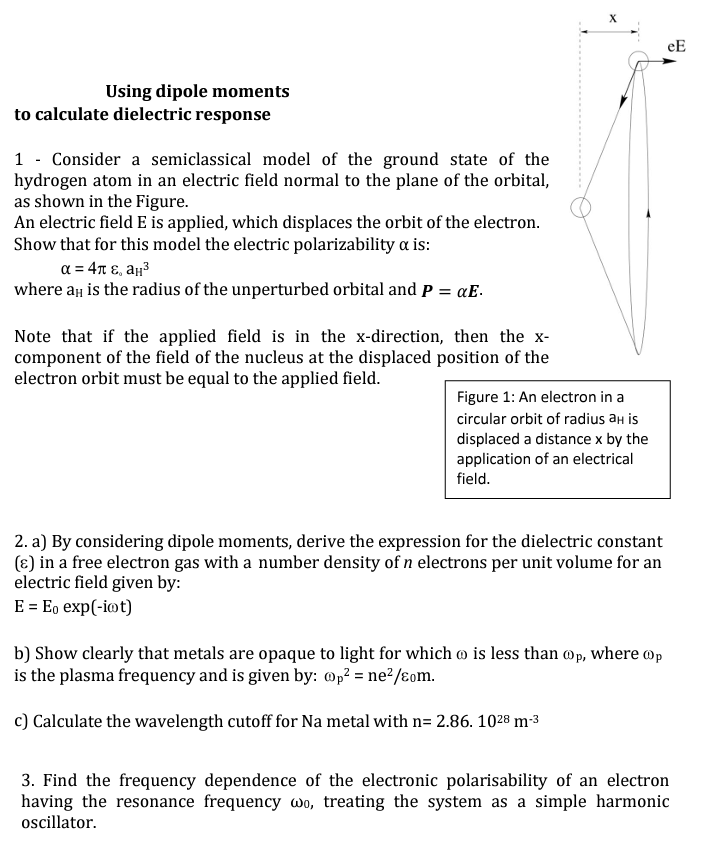
to calculate dielectric response 1 - Consider a semiclassical model of the ground state of the hydrogen atom in an electric field normal to the plane of the orbital, as shown in the Figure. An electric field \\( \\mathrm{E} \\) is applied, which displaces the orbit of the electron. Show that for this model the electric polarizability \\( \\alpha \\) is: \\[ \\alpha=4 \\pi \\varepsilon_{0} \\mathrm{a}_{\\mathrm{H}}{ }^{3} \\] where \\( \\mathrm{a}_{\\mathrm{H}} \\) is the radius of the unperturbed orbital and \\( \\boldsymbol{P}=\\alpha \\boldsymbol{E} \\). Note that if the applied field is in the \\( \\mathrm{x} \\)-direction, then the \\( \\mathrm{x} \\) component of the field of the nucleus at the displaced position of the electron orbit must be equal to the applied field. Figure 1: An electron in a circular orbit of radius \\( \\mathrm{a}_{\\mathrm{H}} \\) is displaced a distance \\( x \\) by the application of an electrical field. 2. a) By considering dipole moments, derive the expression for the dielectric constant \\( (\\varepsilon) \\) in a free electron gas with a number density of \\( n \\) electrons per unit volume for an electric field given by: \\[ \\mathrm{E}=\\mathrm{E}_{0} \\exp (-\\mathrm{i} \\omega \\mathrm{t}) \\] b) Show clearly that metals are opaque to light for which \\( \\omega \\) is less than \\( \\omega_{p} \\), where \\( \\omega_{p} \\) is the plasma frequency and is given by: \\( \\omega_{\\mathrm{p}}^{2}=\\mathrm{ne}^{2} / \\varepsilon_{0} \\mathrm{~m} \\). c) Calculate the wavelength cutoff for Na metal with \\( \\mathrm{n}=2.86 .10^{28} \\mathrm{~m}^{-3} \\) 3. Find the frequency dependence of the electronic polarisability of an electron having the resonance frequency \\( \\omega_{0} \\), treating the system as a simple harmonic oscillator.