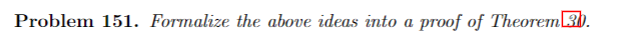(Solved): Theorem 30. (Extreme Value Theorem (EVT)) Suppose \( f \) is continuous on \( [a, b] \). Then ther ...

Theorem 30. (Extreme Value Theorem (EVT)) Suppose \( f \) is continuous on \( [a, b] \). Then there exists \( c, d \in[a, b] \) such that \( f(d) \leq f(x) \leq f(c) \), for all \( x \in[a, b] \) Sketch of Proof: We will first show that \( f \) attains its maximum. To this end, recall that Theorem 2 tells us that \( f[a, b]=\{f(x) \mid x \in[a, b]\} \) is a bounded set. By the LUBP, \( f[a, b] \) must have a least upper bound which we will label \( s \), so that \( s=\sup f[a, b] \). This says that \( s \geq f(x) \), for all \( x \in[a, b] \). All we need to do now is find a \( c \in[a, b] \) with \( f(c)=s \). With this in mind, notice that since \( s=\sup f[a, b] \), then for any positive integer \( n, s-\frac{1}{n} \) is not an upper bound of \( f[a, b] \). Thus there exists \( x_{n} \in[a, b] \) with \( s-\frac{1}{n}