(Solved): The Z Distribution pictured above is also known as the Standard Normal Distribution and first refer ...

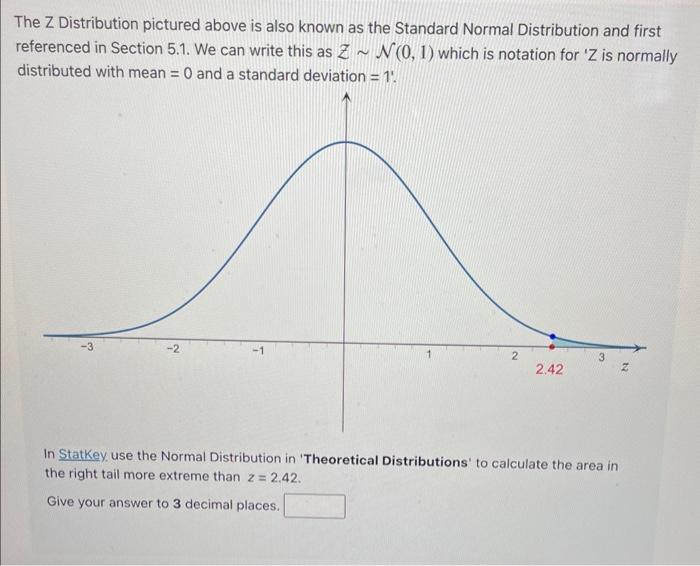
The Z Distribution pictured above is also known as the Standard Normal Distribution and first referenced in Section 5.1. We can write this as \( \mathcal{Z} \sim \mathcal{N}(0,1) \) which is notation for ' \( Z \) is normally distributed with mean \( =0 \) and a standard deviation \( =1 \) : In StatKey, use the Normal Distribution in 'Theoretical Distributions' to calculate the area in the right tail more extreme than \( z=2.42 \). Give your answer to 3 decimal places. The Z Distribution pictured below is also known as the Standard Normal Distribution and first referenced in Section 5.1. We can write this as \( \mathcal{Z} \sim \mathcal{N}(0,1) \) which is notation for ' \( Z \) is normally distributed with mean \( =0 \) and a standard deviation \( =1 \). In StatKey, use the Normal Distribution in 'Theoretical Distributions' to calculate the area in the two tails more extreme than \( z=-2.11 \) \& \( z=2.11 \). Give your answer to 2 decimal places: