(Solved): The total mechanical energy of a (dissipationless) simple harmonic oscillator (a mass \( m \) suspe ...

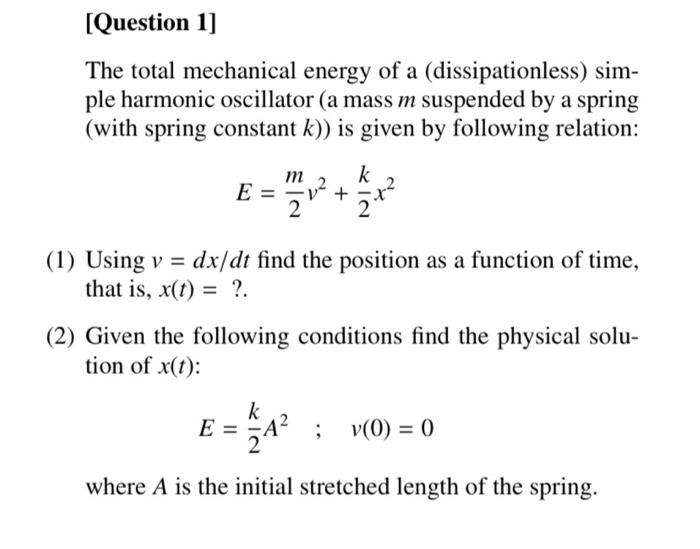
The total mechanical energy of a (dissipationless) simple harmonic oscillator (a mass \( m \) suspended by a spring (with spring constant \( k \) )) is given by following relation: \[ E=\frac{m}{2} v^{2}+\frac{k}{2} x^{2} \] 1) Using \( v=d x / d t \) find the position as a function of time, that is, \( x(t)= \) ?. 2) Given the following conditions find the physical solution of \( x(t) \) : \[ E=\frac{k}{2} A^{2} \quad ; \quad v(0)=0 \] where \( A \) is the initial stretched length of the spring.