(Solved): The toge fied for a poputation P modeled oy dP/dt=3P2P3 is shown in the figure below (a) On a pr ...

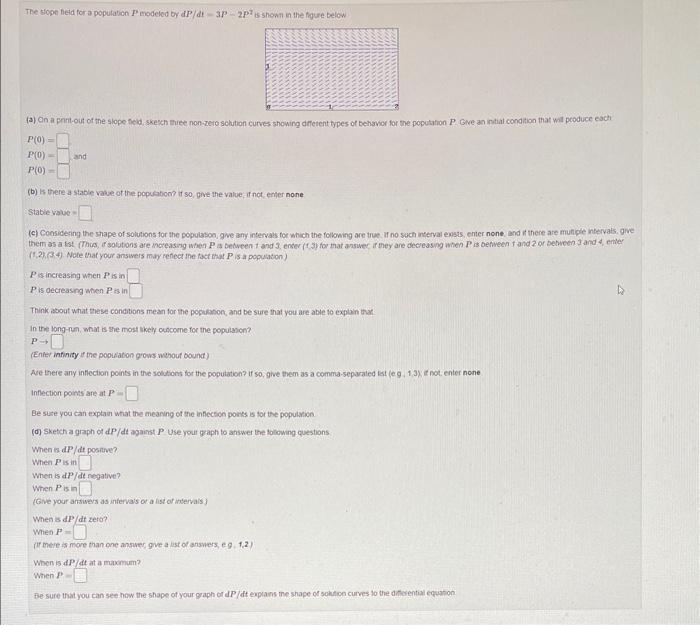
The toge fied for a poputation $P$ modeled oy $dP/dt=3P?2P^3$ is shown in the figure below (a) On a prit-out of the slope feld, skesch thiee non-zero solution cutves showing dffetent types of behswor for the poputation $P$ Gwe an intial condation that wat peoduce each $\begin{array}{l}P(0)=\\ P(0)=?\\ P(0)=\end{array}$ (b) is there a stabie vasue ot the populatoon if so, give the value; if not enter none stabie vaue = (c) Consideang the shape of solutions for the populason;give anny intervals foc which the folowing are true. If no sach inserval ensts, enter none and it there are muniple inteivats. grve them as a tst (This, if solitions are noreisig when $P$ is between t and 3 , enter (f 3 f for that answer if they are deereasing when $P$ is behiveen $f$ and 7 or behicen 3 and 4 , enter (1,2),34) Nole that your answers may refect the fact that $P$ is a popuition) $P$ is increasing when $P$ is in $P$ is aecreasing atsen $P$ is in Think about what thase conditons mean for the poptition, and be sure that you are able to explan thax In the lond ine, what is the most thely cubcome for the fopulason? $P?$ (Enter infinity it the popudabon grows whout bound) Ave there any infiection points in the solutions for the poputation? if so, give them as a comma-sepuraled ist (eg. 13 I i nol, enter none Inflection poins are at $P$ iin Be suse you can exptain what the meaning of the infecson poins is tor the population. (d) Sketch a graph of $dP$ /dt against $P$ Use rout graph to answer the lollowing questions When is $dP/dt$ positive? When $P$ is in When is dP/decnegative? When $P$ is in (Give your amsisers as infervals or a fist of indervals ) When is $dP/dt$ zero? Whed $P^2=$ (If mere is more than one answer, gve a list of answers, eg, 1,2 ) When is dP/at at a maxmum? When $f$ Be sure that you can see how the shape of your graph of $dP/dt$ explans the shape of sohton curves 10 the difcrential equahion.