(Solved): The stress transformation equations can be expressed as: \[ \sigma_{x^{\prime}}-\frac{\sigma_{x}+\s ...

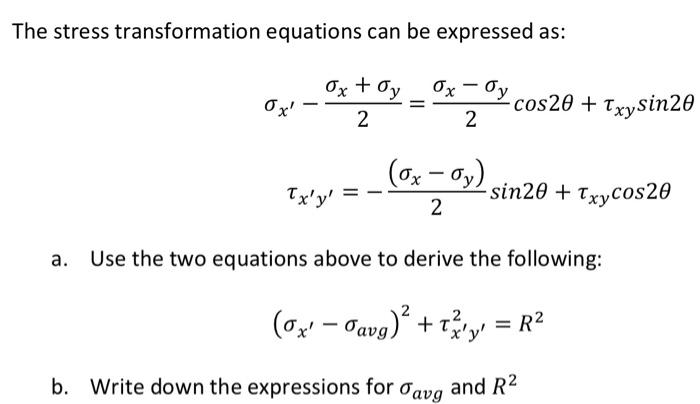
The stress transformation equations can be expressed as: \[ \sigma_{x^{\prime}}-\frac{\sigma_{x}+\sigma_{y}}{2}=\frac{\sigma_{x}-\sigma_{y}}{2} \cos 2 \theta+\tau_{x y} \sin 2 \theta \] \[ \tau_{x^{\prime} y^{\prime}}=-\frac{\left(\sigma_{x}-\sigma_{y}\right)}{2} \sin 2 \theta+\tau_{x y} \cos 2 \theta \] a. Use the two equations above to derive the following: \[ \left(\sigma_{x^{\prime}}-\sigma_{a v g}\right)^{2}+\tau_{x^{\prime} y^{\prime}}^{2}=R^{2} \] b. Write down the expressions for \( \sigma_{a v g} \) and \( R^{2} \)