(Solved): The steady, laminar boundary layer over a fat plate can be stabilized at constant thickness by suc ...

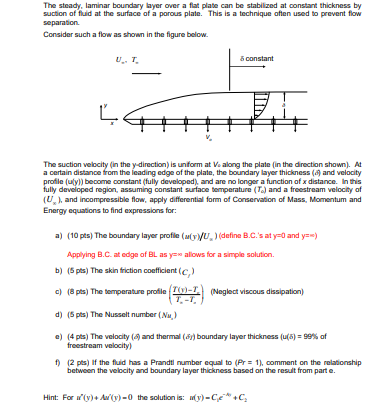
The steady, laminar boundary layer over a fat plate can be stabilized at constant thickness by suction of fluid at the surface of a porous plate. This is a technique aften used to prevent flow eeparation. Consider such a flow as shown in the figure below. The suction velocity (in the $$y$$-direction) is uniorm at $$Va$$ along the plate (in the direction shawn). At a certain distance from the ieading edge of the plate, the boundary layer thickness (d) and velocity profie (ufy)) become constant (fully developed), and are no longer a function of $$x$$ distance. In this fully developed region, assuming constant surface temperature $$(T_b$$ ) and a freestream velocity af $$\left(U_{\mathrm{x}}\right)$$, and incompressible flow, apply differential form of Conservation of Mass, Momentum and Energy equatons to find expressions for: a) (10 pts) The boundary layer profle $$\left(u(r)/U_{?}\right)$$ (define B.C. 's at $$y=0$$ and $$y==$$ ) Applying B.C. at edge of BL as y=w allows for a simple solution. b) (5 pts) The skin friction coefficient $$\left(C_C\right)$$ c) (8 pts) The temperature protile $$\left(\frac{T(y)?T_v}{T_n?T_n}\right)$$ (Neglect viscous dissipation) d) (5 pts) The Nusselt number ( $${\mathrm{Nu}}_{u_2})$$ e) (4 pts) The velocity ( $$($$ ) and thermal ( $$6j)$$ boundary layer thickness (u(s) = ggw of freestream velocity) f) (2 pts) if the fluid has a Prandtl number equal to (Fr $$=1$$ ), comment on the relationship between the velocity and boundary layer thickness based on the result from part e. Hint: For $$x^{??}(y)+A{v}^{?}(y)=0$$ the solution is: $$m(y)=C_1{e}^{?1y}+C_2$$