(Solved): The (standard) Cauchy distribution has a probability density function defined by f(x)=(1+x2)1, ...

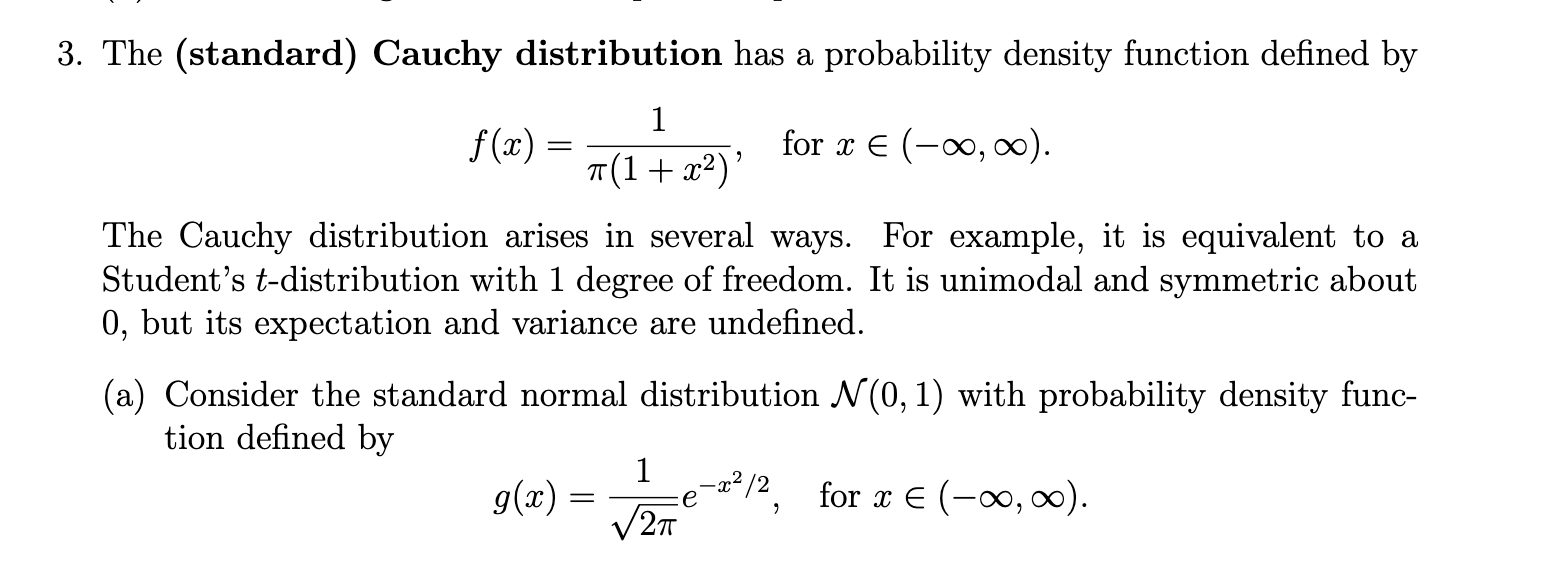
The (standard) Cauchy distribution has a probability density function defined by $f(x)=\frac{1}{?\left(1+x^2\right)},\text{ for }x?(??,?).$ The Cauchy distribution arises in several ways. For example, it is equivalent to a Student's $t$-distribution with 1 degree of freedom. It is unimodal and symmetric about 0 , but its expectation and variance are undefined. 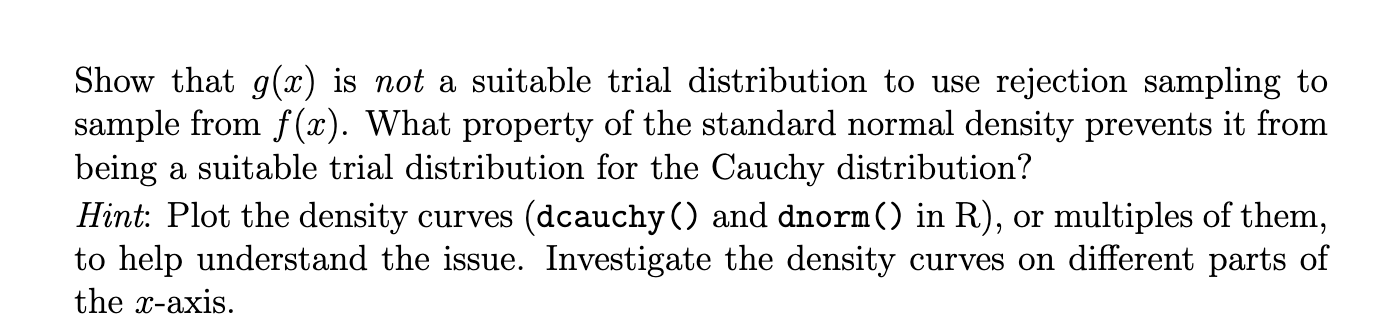(a) Consider the standard normal distribution $\mathcal{N}(0,1)$ with probability density function defined by $g(x)=\frac{1}{\sqrt{2?}}{e}^{?x^2/2},\text{ for }x?(??,?)$ Show that $g(x)$ is not a suitable trial distribution to use rejection sampling to sample from $f(x)$. What property of the standard normal density prevents it from being a suitable trial distribution for the Cauchy distribution? Hint: Plot the density curves (dcauchy () and dnorm() in R), or multiples of them, to help understand the issue. Investigate the density curves on different parts of the $x$-axis. It can be shown that if $U$ and $V$ are independent $\mathcal{N}(0,1)$ random variables, then the ratio $X=U/V$ has a standard Cauchy distribution. This construction allows us to generate samples from the Cauchy distribution. If $X_1,X_2,\dots ,X_n$ are iid standard Cauchy random variables, consider the sample mean $\stackrel{?}{X}=\frac{1}{n}{?}_{i=1}^n{X}_i.$ For $n=100$ and 1000 repetitions, approximate the sampling distribution of the sample mean to show that $\stackrel{?}{X}$ does not have a normal distribution. In particular, standardize the sampling distribution and verify that it is does not resemble a $\mathcal{N}(0,1)$ distribution. Note: This problem shows that the Central Limit Theorem does not apply to the Cauchy distribution. In fact, it can be shown that $\stackrel{?}{X}$ itself has a standard Cauchy distribution.