(Solved): The probability of symbol error for coherent M-ary PSK in an additive white Gaussian noise environ ...

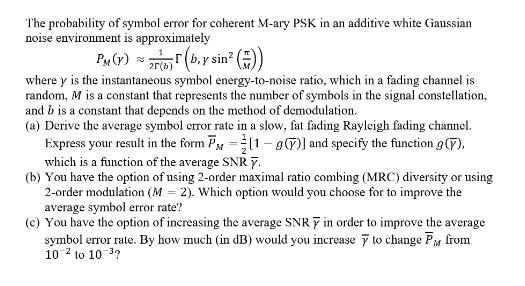
The probability of symbol error for coherent M-ary PSK in an additive white Gaussian noise environment is approximately $P_M(?)?\frac{1}{2?(b)}?\left(b,?{\sin }^2\left(\frac{?}{M}\right)\right)$ where $?$ is the instantaneous symbol energy-to-noise ratio, which in a fading channel is random, $M$ is a constant that represents the number of symbols in the signal constellation, and $b$ is a constant that depends on the method of demodulation. (a) Derive the average symbol error rate in a slow, fat fading Rayleigh fading channel. Express your result in the form ${\stackrel{?}{P}}_M=\frac{1}{2}[1?g(\stackrel{?}{?})]$ and specify the function $g(\stackrel{?}{?})$, which is a function of the average $\mathrm{SNR}\stackrel{?}{?}$. (b) You have the option of using 2-order maximal ratio combing (MRC) diversity or using 2-order modulation $(M=2)$. Which option would you choose for to improve the average symbol error rate? (c) You have the option of increasing the average SNR $\stackrel{?}{?}$ in order to improve the average symbol error rate. By how much (in dB) would you increase $\stackrel{?}{?}$ to change ${\stackrel{?}{P}}_M$ from $10^{?2}$ to $10^{?3}$ ?