(Solved): The octagonal slab shown in Figure Q4 has side length \( 1.66 \mathrm{~m} \), and is supported on ...

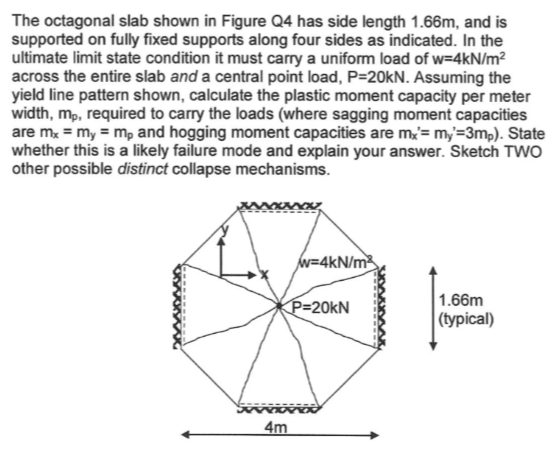
The octagonal slab shown in Figure Q4 has side length \( 1.66 \mathrm{~m} \), and is supported on fully fixed supports along four sides as indicated. In the ultimate limit state condition it must carry a uniform load of \( w=4 \mathrm{kN} / \mathrm{m}^{2} \) across the entire slab and a central point load, \( P=20 \mathrm{kN} \). Assuming the yield line pattern shown, calculate the plastic moment capacity per meter width, \( m_{p} \), required to carry the loads (where sagging moment capacities are \( m_{x}=m_{y}=m_{p} \) and hogging moment capacities are \( m_{x}^{\prime}=m_{y}{ }^{\prime}=3 m_{p} \) ). State whether this is a likely failure mode and explain your answer. Sketch TWO other possible distinct collapse mechanisms.