(Solved): The normalized wave function of a hydogen-like atom is given by \[ \psi_{n \ell}^{m}(r, \theta, \ph ...

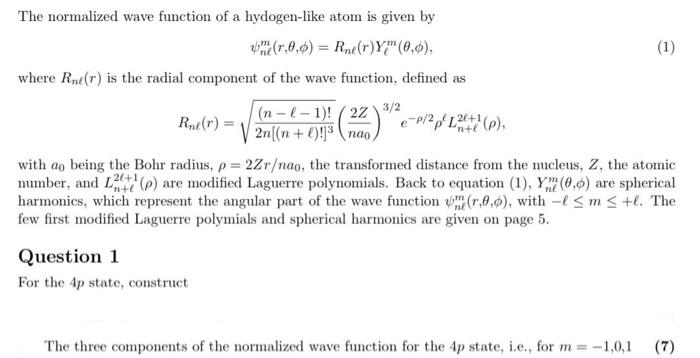
The normalized wave function of a hydogen-like atom is given by \[ \psi_{n \ell}^{m}(r, \theta, \phi)=R_{n \ell}(r) Y_{\ell}^{m}(\theta, \phi), \] where \( R_{n \ell}(r) \) is the radial component of the wave function, defined as \[ R_{n \ell}(r)=\sqrt{\frac{(n-\ell-1) !}{2 n[(n+\ell) !]^{3}}}\left(\frac{2 Z}{n a_{0}}\right)^{3 / 2} e^{-\rho / 2} \rho^{\ell} L_{n+\ell}^{2 \ell+1}(\rho), \] with \( a_{0} \) being the Bohr radius, \( \rho=2 Z r / n a_{0} \), the transformed distance from the nucleus, \( Z \), the atomic number, and \( L_{n+\ell}^{2 \ell+1}(\rho) \) are modified Laguerre polynomials. Back to equation (1), \( Y_{n \ell}^{m}(\theta, \phi) \) are spherical harmonics, which represent the angular part of the wave function \( \psi_{n \ell}^{m}(r, \theta, \phi) \), with \( -\ell \leq m \leq+\ell \). The few first modified Laguerre polymials and spherical harmonics are given on page 5. Question 1 For the \( 4 p \) state, construct The three components of the normalized wave function for the \( 4 p \) state, i.e., for \( m=-1,0,1 \)