(Solved): The NACA 4412 airfoil has a mean camber line given by \[ \frac{z}{c}=\left\{\begin{array}{ll} 0.25\ ...

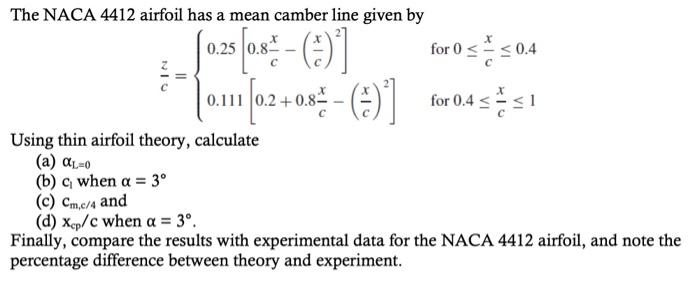
The NACA 4412 airfoil has a mean camber line given by \[ \frac{z}{c}=\left\{\begin{array}{ll} 0.25\left[0.8 \frac{x}{c}-\left(\frac{x}{c}\right)^{2}\right] & \text { for } 0 \leq \frac{x}{c} \leq 0.4 \\ 0.111\left[0.2+0.8 \frac{x}{c}-\left(\frac{x}{c}\right)^{2}\right] & \text { for } 0.4 \leq \frac{x}{c} \leq 1 \end{array}\right. \] Using thin airfoil theory, calculate (a) \( \alpha_{\mathrm{L}=0} \) (b) \( c_{1} \) when \( \alpha=3^{\circ} \) (c) \( \mathrm{c}_{m, c / 4} \) and (d) \( \mathrm{x}_{\mathrm{cp}} / \mathrm{c} \) when \( \alpha=3^{\circ} \). Finally, compare the results with experimental data for the NACA 4412 airfoil, and note the percentage difference between theory and experiment.