(Solved): The MacLaurin series expansion for \( \cos x \) is \[ \cos x=1-\frac{x^{2}}{2}+\frac{x^{4}}{4 !}-\ ...

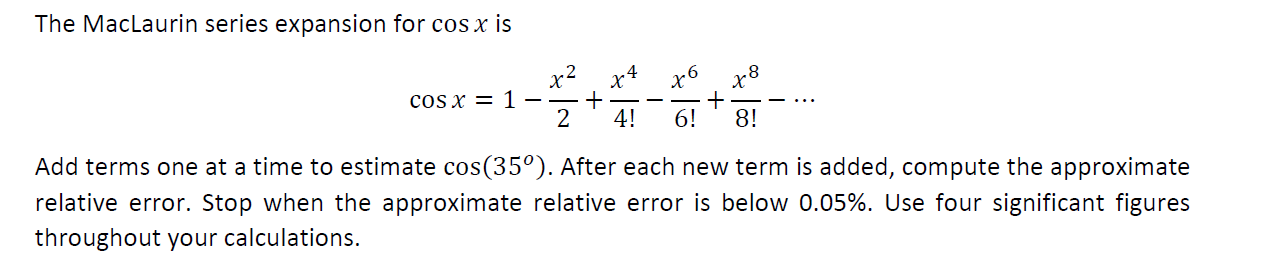
The MacLaurin series expansion for \( \cos x \) is \[ \cos x=1-\frac{x^{2}}{2}+\frac{x^{4}}{4 !}-\frac{x^{6}}{6 !}+\frac{x^{8}}{8 !}-\cdots \] Add terms one at a time to estimate \( \cos \left(35^{\circ}\right) \). After each new term is added, compute the approximate relative error. Stop when the approximate relative error is below \( 0.05 \% \). Use four significant figures throughout your calculations.