(Solved): The general form for the inductance of a solenoid (a long, straight coil of wire) is = N^2A/l ...

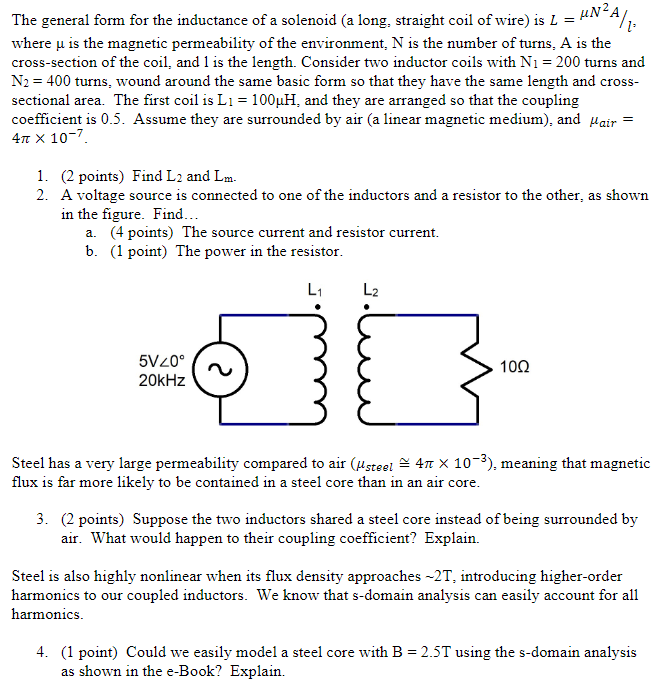
The general form for the inductance of a solenoid (a long, straight coil of wire) is \\( L=\\mu N^{2} \\mathrm{~A} / l \\), where \\( \\mu \\) is the magnetic permeability of the environment, \\( N \\) is the number of turns, \\( A \\) is the cross-section of the coil, and 1 is the length. Consider two inductor coils with \\( \\mathrm{N}_{1}=200 \\) turns and \\( \\mathrm{N}_{2}=400 \\) turns, wound around the same basic form so that they have the same length and crosssectional area. The first coil is \\( \\mathrm{L}_{1}=100 \\mu \\mathrm{H} \\), and they are arranged so that the coupling coefficient is 0.5 . Assume they are surrounded by air (a linear magnetic medium), and \\( \\mu_{\\text {air }}= \\) \\( 4 \\pi \\times 10^{-7} \\). 1. (2 points) Find \\( \\mathrm{L}_{2} \\) and \\( \\mathrm{L}_{\\mathrm{m}} \\). 2. A voltage source is connected to one of the inductors and a resistor to the other, as shown in the figure. Find... a. (4 points) The source current and resistor current. b. (1 point) The power in the resistor. Steel has a very large permeability compared to air \\( \\left(\\mu_{\\text {steel }} \\cong 4 \\pi \\times 10^{-3}\\right) \\), meaning that magnetic flux is far more likely to be contained in a steel core than in an air core. 3. (2 points) Suppose the two inductors shared a steel core instead of being surrounded by air. What would happen to their coupling coefficient? Explain. Steel is also highly nonlinear when its flux density approaches \\( \\sim 2 \\mathrm{~T} \\), introducing higher-order harmonics to our coupled inductors. We know that s-domain analysis can easily account for all harmonics. 4. (1 point) Could we easily model a steel core with \\( \\mathrm{B}=2.5 \\mathrm{~T} \\) using the \\( \\mathrm{s} \\)-domain analysis as shown in the e-Book? Explain.