(Solved): The following differential equation with initial conditions is a mass-spring-damper system. Mdx ...

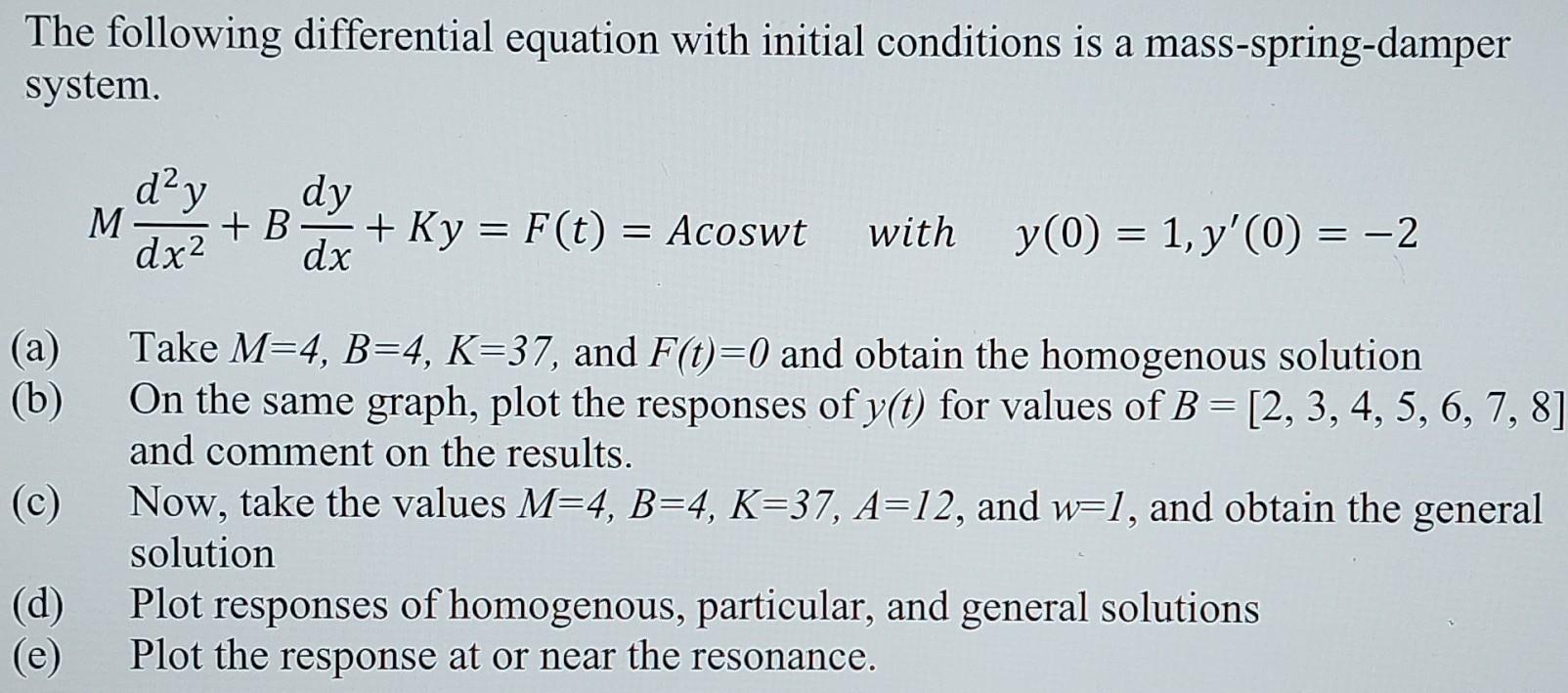
The following differential equation with initial conditions is a mass-spring-damper system. $$M\frac{d^{2}y}{d{x}^2}+B\frac{dy}{dx}+Ky=F(t)=A\cos wt\text{ with }y(0)=1,y^{?}(0)=?2$$ (a) Take $$M=4,B=4,K=37$$, and $$F(t)=0$$ and obtain the homogenous solution (b) On the same graph, plot the responses of $$y(t)$$ for values of $$B=[2,3,4,5,6,7,8]$$ and comment on the results. (c) Now, take the values $$M=4,B=4,K=37,A=12$$, and $$w=1$$, and obtain the general solution (d) Plot responses of homogenous, particular, and general solutions (e) Plot the response at or near the resonance.