(Solved): The figure shows the flow of traffic (in vehicles per hour) through a network of streets. (a) Solve ...

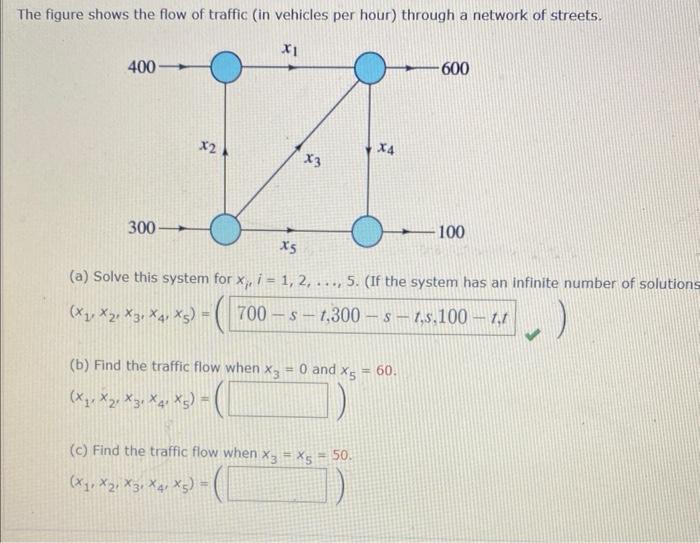
The figure shows the flow of traffic (in vehicles per hour) through a network of streets. (a) Solve this system for \( x_{i}, i=1,2, \ldots, 5 \). (If the system has an infinite number \( \left(x_{1}, x_{2}, x_{3}, x_{4}, x_{5}\right)=(700-s-t, 300-s-t, s, 100-t, t) \) (b) Find the traffic flow when \( x_{3}=0 \) and \( x_{5}=60 \). \( \left(x_{1}, x_{2}, x_{3}, x_{4}, x_{5}\right)=( \) (c) Find the traffic flow when \( x_{3}=x_{5}=50 \). \( \left(x_{1}, x_{2}, x_{3}, x_{4}, x_{5}\right)= \)