(Solved): The figure shows a point charge with two different Gaussian surfaces that are centered on the c ...

The figure shows a point charge with two different Gaussian surfaces that are centered on the charge. For each surface what is the electric flux through the surface, and can you use symmetry and Gauss’s law to calculate ????????, the electric field at point P. Select all that apply.
A. ????? = ????/????0 for surface 1.
B. ????? = ????/????0 for surface 2.
C. For surface 1, you can calculate ???????? using symmetry and Gauss’s law.
D. For surface 2, you can calculate ???????? using symmetry and Gauss’s law.

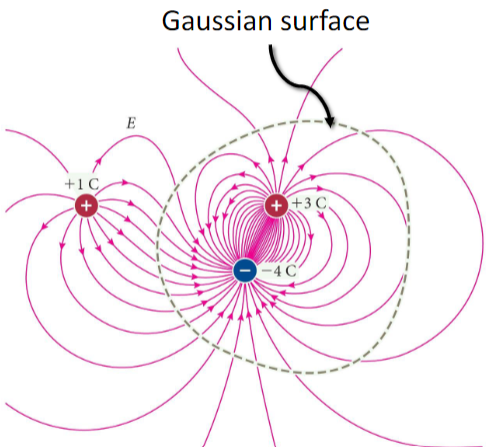
The figure shows field lines for a configuration of charges. If the left charge were removed, select all of the correct statements.
A. The flux through the Gaussian surface would remain the same.
B. The electric field at all points along the Gaussian surface would remain the same.
C. None of the above.

An infinitely long cylindrical rod of radius ????0 has uniform volume charge density ????. Consider coaxial cylindrical Gaussian surfaces in two different cases as shown. What is the ratio of flux through the Gaussian surface in case 1 over the flux through the Gaussian surface in case 2, ?????,1/?????,2?