(Solved): The equation \( x^{2}-x y+y^{2}=3 \) represents an ellipse whose axes are not parallel to the coor ...

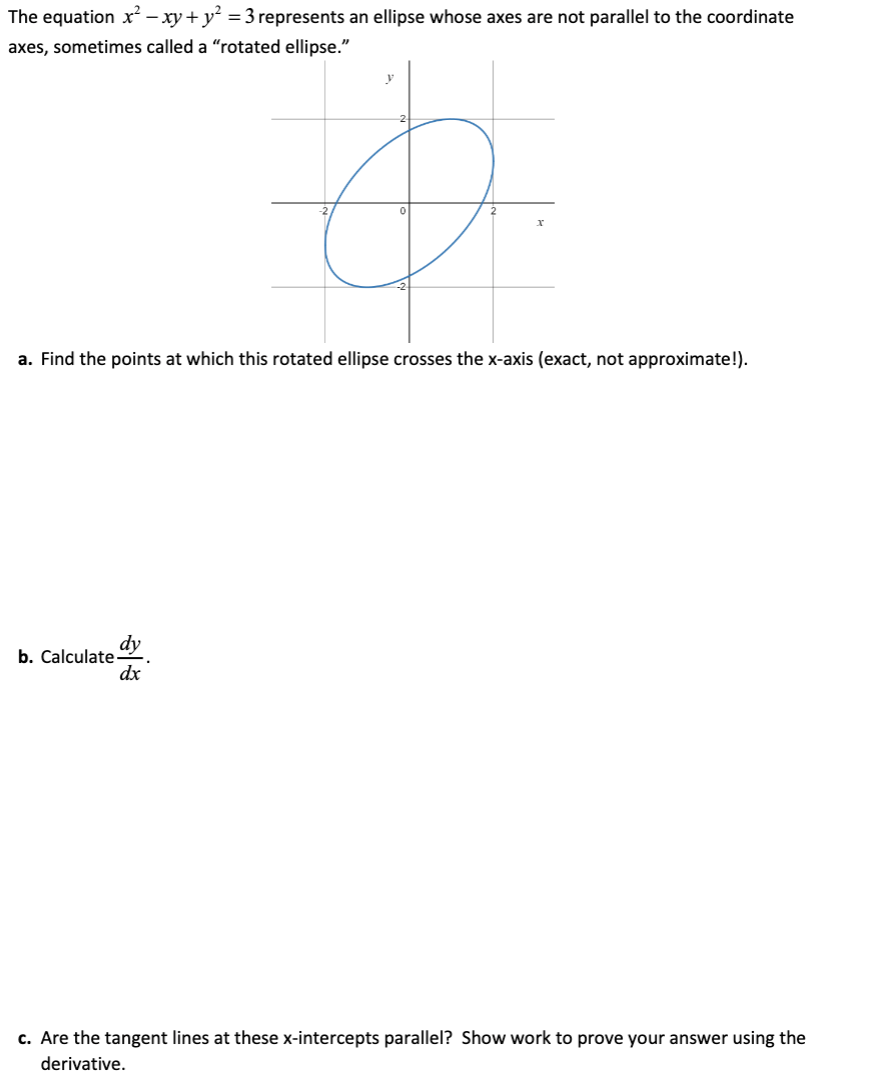
The equation \( x^{2}-x y+y^{2}=3 \) represents an ellipse whose axes are not parallel to the coordinate axes, sometimes called a "rotated ellipse." a. Find the points at which this rotated ellipse crosses the \( x \)-axis (exact, not approximate!). b. Calculate \( \frac{d y}{d x} \). c. Are the tangent lines at these \( x \)-intercepts parallel? Show work to prove your answer using the derivative.