(Solved): The electric field pattern at a point \( (x, y, z=0) \) for a beam propagatin ...

???????

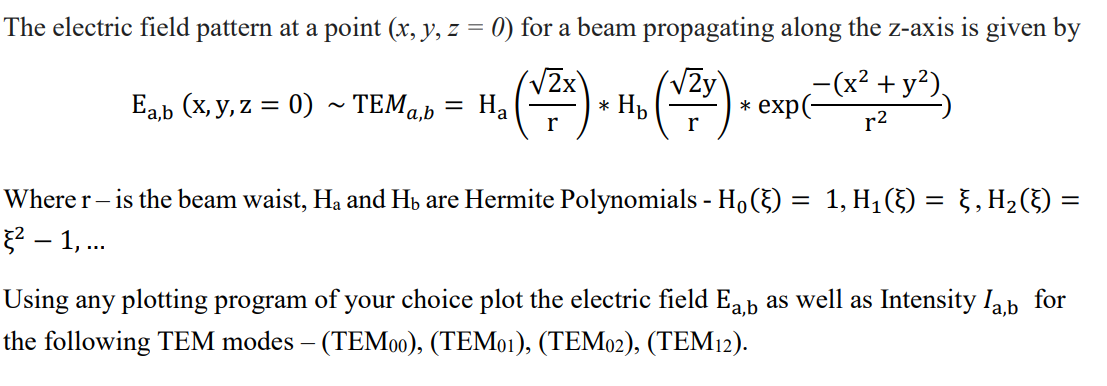
The electric field pattern at a point \( (x, y, z=0) \) for a beam propagating along the \( \mathrm{z} \)-axis is given by \[ \mathrm{E}_{\mathrm{a}, \mathrm{b}}(\mathrm{x}, \mathrm{y}, \mathrm{z}=0) \sim \mathrm{TE} M_{a, b}=\mathrm{H}_{\mathrm{a}}\left(\frac{\sqrt{2} \mathrm{x}}{\mathrm{r}}\right) * \mathrm{H}_{\mathrm{b}}\left(\frac{\sqrt{2} \mathrm{y}}{\mathrm{r}}\right) * \exp \left(\frac{-\left(\mathrm{x}^{2}+\mathrm{y}^{2}\right)}{\mathrm{r}^{2}}\right) \] Where \( \mathrm{r} \) - is the beam waist, \( \mathrm{H}_{\mathrm{a}} \) and \( \mathrm{H}_{\mathrm{b}} \) are Hermite Polynomials \( -\mathrm{H}_{0}(\xi)=1, \mathrm{H}_{1}(\xi)=\xi, \mathrm{H}_{2}(\xi)= \) \( \xi^{2}-1, \ldots \) Using any plotting program of your choice plot the electric field \( \mathrm{E}_{\mathrm{a}, \mathrm{b}} \) as well as Intensity \( I_{\mathrm{a}, \mathrm{b}} \) for