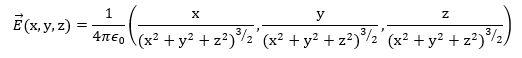(Solved): The electric field of a charge of 1 C, located at the point (0,0,0), is given by the following exp ...

The electric field of a charge of 1 ?C, located at the point (0,0,0), is given by the following expression.

Where  It is the electrical permittivity of vacuum. Taking into account the above:

a)  What methods do you know to determine the electrical potential?

b)  Argue the choice of one of the methods and determine the electric potential at a point (x, y, z)?0

 

Note: One way to calculate the electric potential is through an integral path/line. If you consider a radial path from infinity to point (x, y, z), the line differential is d=(dx, dy, dz). If you use this information, argue why the integral is taken from (x, y, z) ? (?, ?, ?) and not from the coordinate origin (0,0,0).

\( \vec{E}(\mathrm{x}, \mathrm{y}, \mathrm{z})=\frac{1}{4 \pi \epsilon_{0}}\left(\frac{\mathrm{x}}{\left(\mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}\right)^{3 / 2}}, \frac{\mathrm{y}}{\left(\mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}\right)^{3 / 2}}, \frac{\mathrm{z}}{\left(\mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}\right)^{3 / 2}}\right) \)