(Solved): The Electric Field Between Two Parallel Plates of Opposite Charge relative to the plate dimensions ...

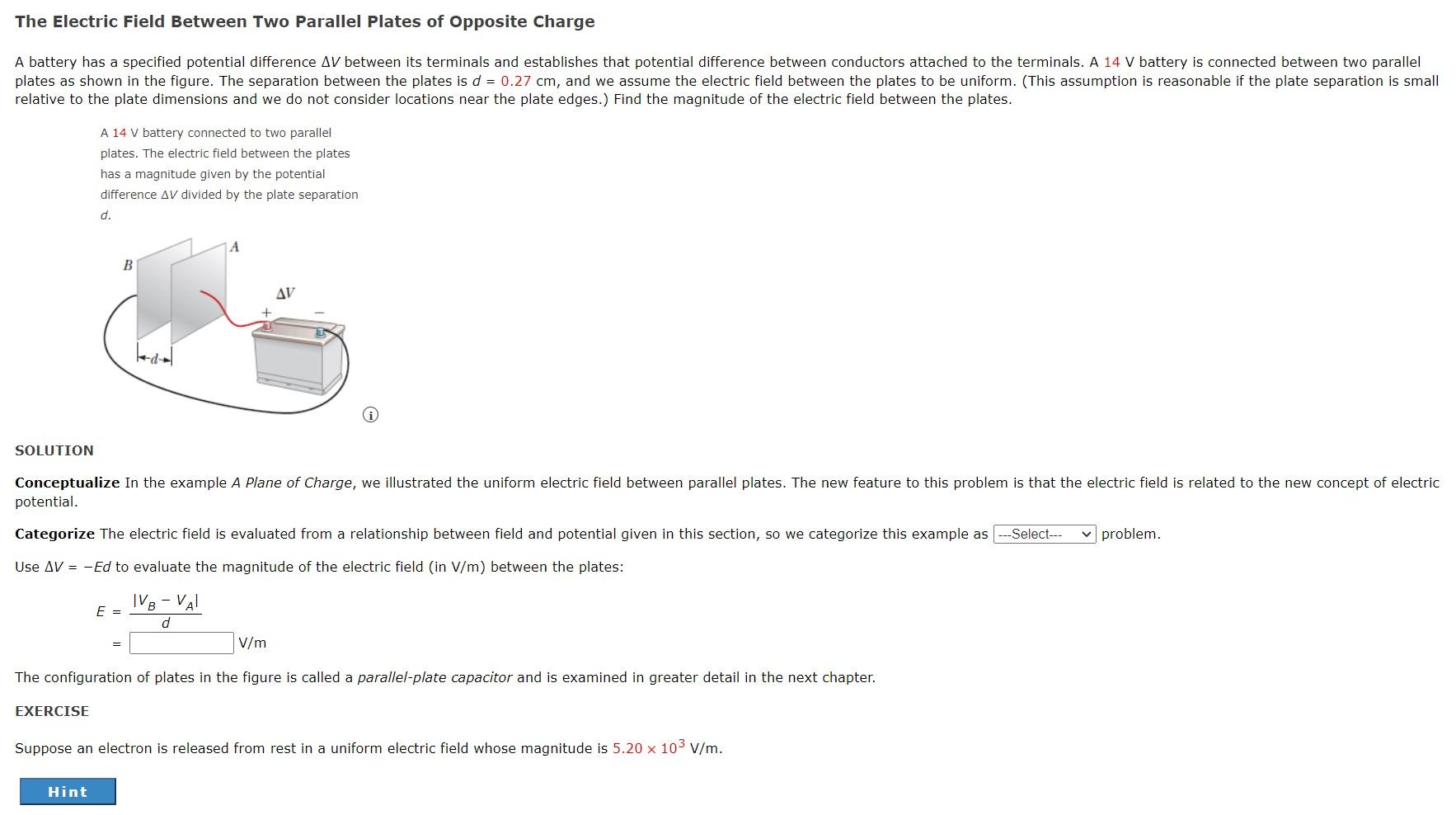
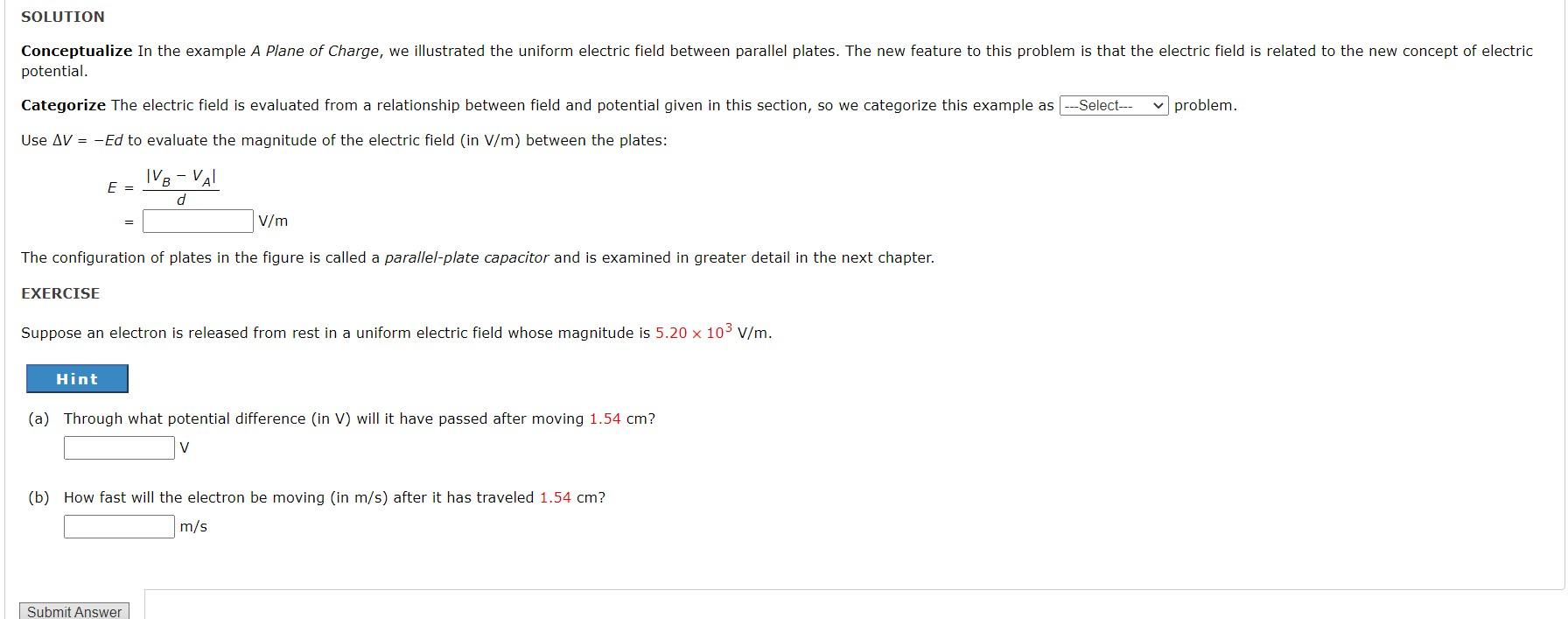
The Electric Field Between Two Parallel Plates of Opposite Charge relative to the plate dimensions and we do not consider locations near the plate edges.) Find the magnitude of the electric field between the plates. A \( 14 \mathrm{~V} \) battery connected to two parallel plates. The electric field between the plates has a magnitude given by the potential difference \( \Delta V \) divided by the plate separation d. SOLUTION potential. Categorize The electric field is evaluated from a relationship between field and potential given in this section, so we categorize this example as problem. Use \( \Delta V=-E d \) to evaluate the magnitude of the electric field (in \( \mathrm{V} / \mathrm{m} \) ) between the plates: \( E=\frac{\left|V_{B}-V_{A}\right|}{d} \) \( =\quad \mathrm{V} / \mathrm{m} \) The configuration of plates in the figure is called a parallel-plate capacitor and is examined in greater detail in the next chapter. EXERCISE Suppose an electron is released from rest in a uniform electric field whose magnitude is \( 5.20 \times 10^{3} \mathrm{~V} / \mathrm{m} \). potential. Use \( \Delta V=-E d \) to evaluate the magnitude of the electric field (in \( \mathrm{V} / \mathrm{m} \) ) between the plates: \( E=\frac{\left|V_{B}-V_{A}\right|}{d} \) \( =\mathrm{V} / \mathrm{m} \) The configuration of plates in the figure is called a parallel-plate capacitor and is examined in greater detail in the next chapter. EXERCISE Suppose an electron is released from rest in a uniform electric field whose magnitude is \( 5.20 \times 10^{3} \mathrm{~V} / \mathrm{m} \). (a) Through what potential difference (in V) will it have passed after moving \( 1.54 \mathrm{~cm} \) ? \( \mathrm{v} \) (b) How fast will the electron be moving (in \( \mathrm{m} / \mathrm{s} \) ) after it has traveled \( 1.54 \mathrm{~cm} \) ? \( \mathrm{m} / \mathrm{s} \)