(Solved): The cross-sectional dimensions of a beam are shown. Assume \( b_{f}=2.75 \mathrm{in} ., t_{f}=t_{w} ...

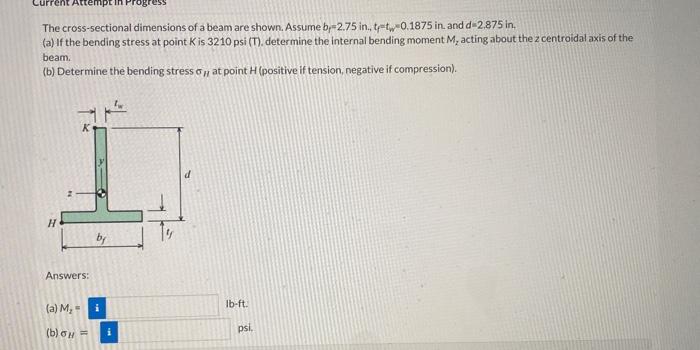
The cross-sectional dimensions of a beam are shown. Assume \( b_{f}=2.75 \mathrm{in} ., t_{f}=t_{w}=0.1875 \mathrm{in} \). and \( d=2.875 \mathrm{in} \), (a) If the bending stress at point \( K \) is 3210 psi (T). determine the internal bending moment \( M_{z} \) acting about the z centroidal axis of the beam. (b) Determine the bending stress \( \sigma_{H} \) at point \( H \) (positive if tension, negative if compression). Answers: (a) \( M_{2}= \) \( \mathrm{Ib} \cdot \mathrm{ft} \). (b) \( \sigma_{H}= \) psi.