(Solved): The columns of \( Q \) were obtained by applying the Gram-Schmidt process to the columns of \( A \) ...

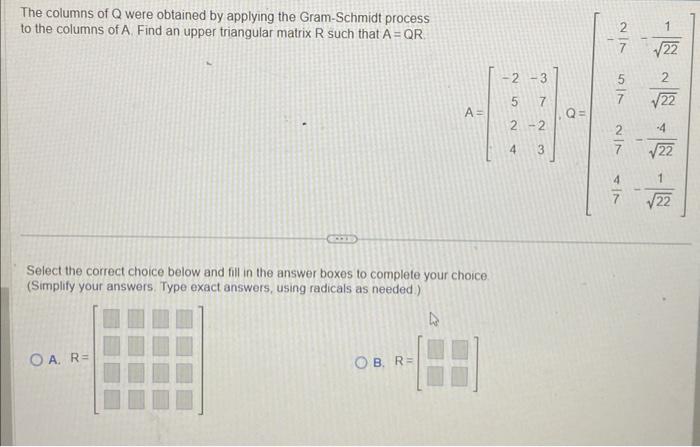
The columns of \( Q \) were obtained by applying the Gram-Schmidt process to the columns of \( A \). Find an upper triangular matrix \( R \) such that \( A=Q R \) \[ A=\left[\begin{array}{rr} -2 & -3 \\ 5 & 7 \\ 2 & -2 \\ 4 & 3 \end{array}\right], Q=\left[\begin{array}{rr} -\frac{2}{7} & -\frac{1}{\sqrt{22}} \\ \frac{5}{7} & \frac{2}{\sqrt{22}} \\ \frac{2}{7} & -\frac{4}{\sqrt{22}} \\ \frac{4}{7} & -\frac{1}{\sqrt{22}} \end{array}\right] \] Select the correct choice below and fill in the answer boxes to complete your choice (Simplify your answers. Type exact answers, using radicals as needed.) A. \( R= \) B. \( R= \)