(Solved): The absorption cross section on the ordinate of the ozone absorption spectrum is defined by Transm ...

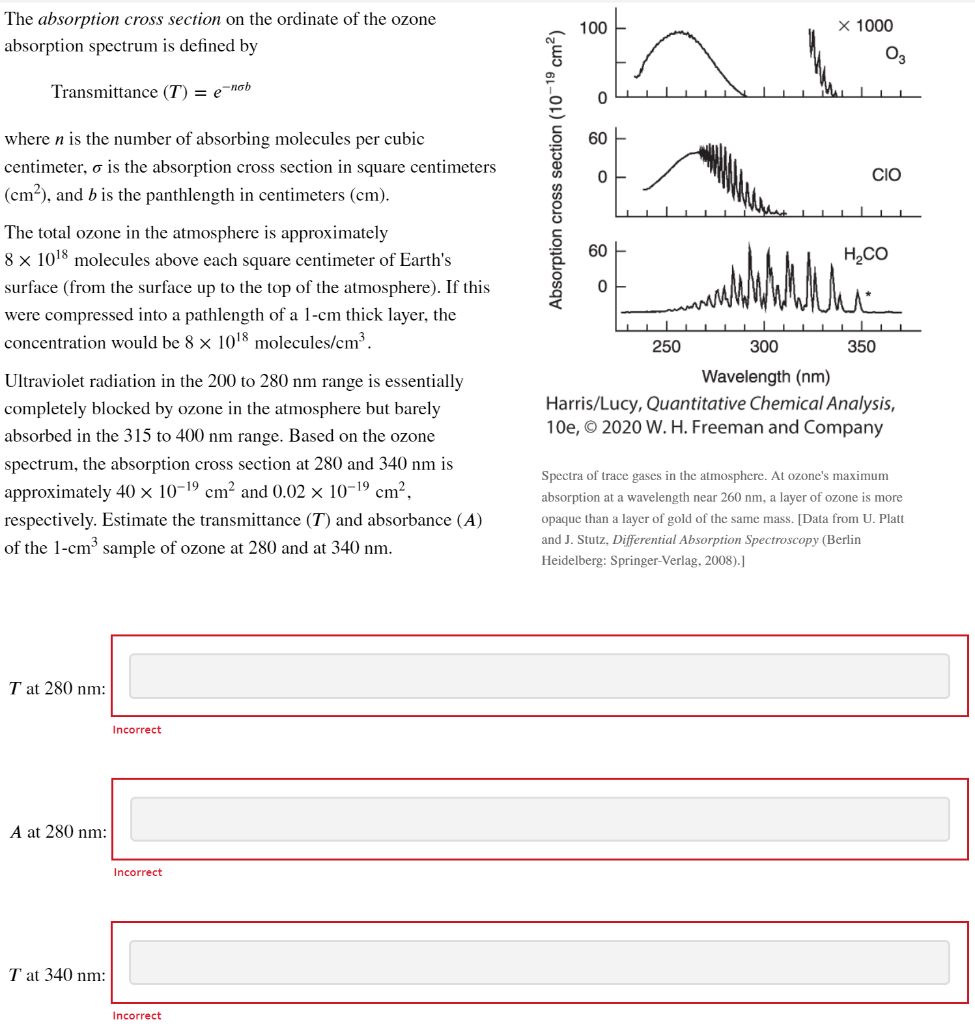
The absorption cross section on the ordinate of the ozone absorption spectrum is defined by Transmittance \( (T)=e^{-n \sigma b} \) where \( n \) is the number of absorbing molecules per cubic centimeter, \( \sigma \) is the absorption cross section in square centimeters \( \left(\mathrm{cm}^{2}\right) \), and \( b \) is the panthlength in centimeters \( (\mathrm{cm}) \). The total ozone in the atmosphere is approximately \( 8 \times 10^{18} \) molecules above each square centimeter of Earth's surface (from the surface up to the top of the atmosphere). If this were compressed into a pathlength of a 1-cm thick layer, the concentration would be \( 8 \times 10^{18} \) molecules \( / \mathrm{cm}^{3} \). Ultraviolet radiation in the 200 to \( 280 \mathrm{~nm} \) range is essentially completely blocked by ozone in the atmosphere but barely Harris/Lucy, Quantitative Chemical Analysis, absorbed in the 315 to \( 400 \mathrm{~nm} \) range. Based on the ozone 10e, @ 2020 W. H. Freeman and Company spectrum, the absorption cross section at 280 and \( 340 \mathrm{~nm} \) is approximately \( 40 \times 10^{-19} \mathrm{~cm}^{2} \) and \( 0.02 \times 10^{-19} \mathrm{~cm}^{2} \), Spectra of trace gases in the atmosphere. At ozone's maximum respectively. Estimate the transmittance \( (T) \) and absorbance \( (A) \quad \) opaque than a layer of gold of the same mass. [Data from U. Platt of the \( 1-\mathrm{cm}^{3} \) sample of ozone at 280 and at \( 340 \mathrm{~nm} \). and J. 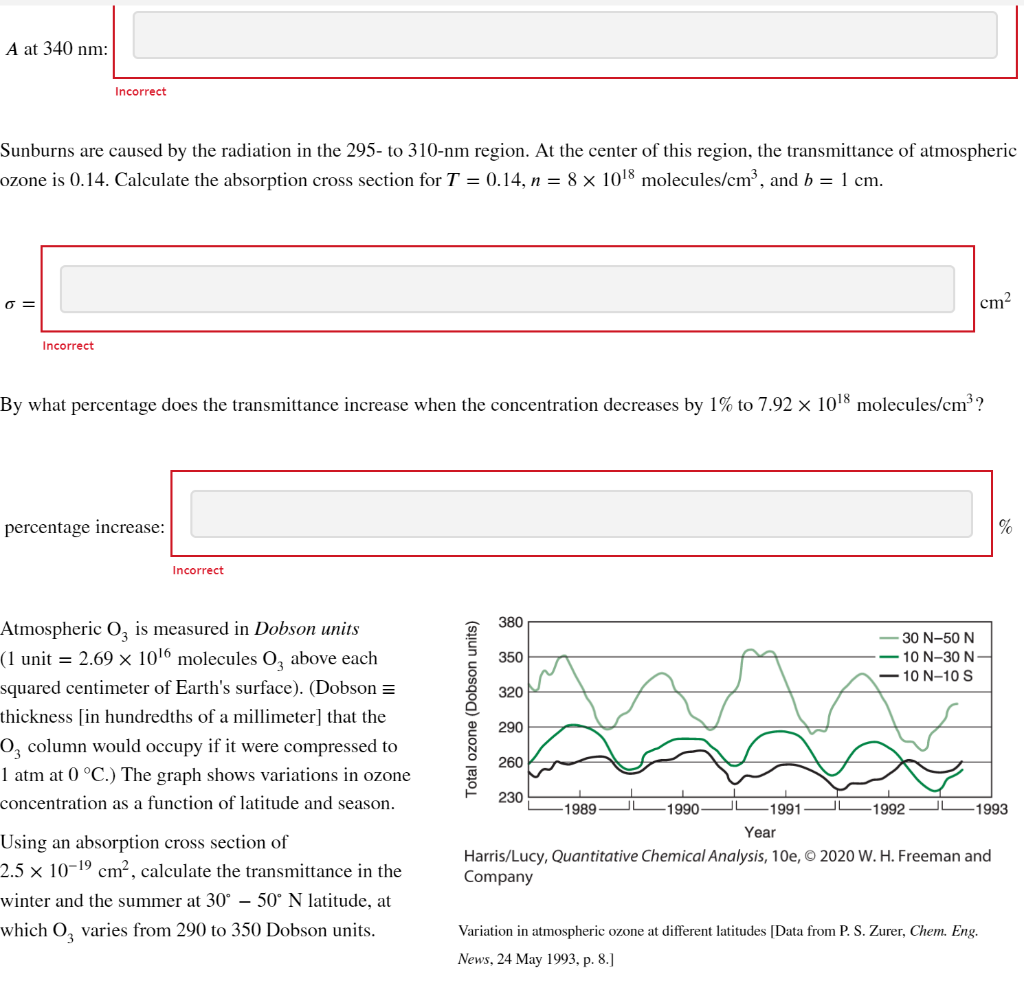Stutz, Differential Absorption Spectroscopy (Berlin Heidelberg: Springer-Verlag, 2008).] \( T \) at \( 280 \mathrm{~nm}: \) Incorrect \( A \) at \( 280 \mathrm{~nm}: \) Incorrect \( T \) at \( 340 \mathrm{~nm}: \) Sunburns are caused by the radiation in the 295-to 310 -nm region. At the center of this region, the transmittance of atmospheric ozone is \( 0.14 \). Calculate the absorption cross section for \( T=0.14, n=8 \times 10^{18} \) molecules \( / \mathrm{cm}^{3} \), and \( b=1 \mathrm{~cm} \). \( \sigma \) Incorrect By what percentage does the transmittance increase when the concentration decreases by \( 1 \% \) to \( 7.92 \times 10^{18} \) molecules/cm \( { }^{3} \) ? percentage increase: Incorrect Atmospheric \( \mathrm{O}_{3} \) is measured in Dobson units ( 1 unit \( =2.69 \times 10^{16} \) molecules \( \mathrm{O}_{3} \) above each squared centimeter of Earth's surface). (Dobson \( \equiv \) thickness [in hundredths of a millimeter] that the \( \mathrm{O}_{3} \) column would occupy if it were compressed to \( 1 \mathrm{~atm} \) at \( 0^{\circ} \mathrm{C} \).) The graph shows variations in ozone concentration as a function of latitude and season. Using an absorption cross section of \( 2.5 \times 10^{-19} \mathrm{~cm}^{2} \), calculate the transmittance in the Harris/Lucy, Quantitative Chemical Analysis, 10e, @ \( 2020 \mathrm{~W} \). H. Freeman and winter and the summer at \( 30^{\circ}-50^{\circ} \mathrm{N} \) latitude, at which \( \mathrm{O}_{3} \) varies from 290 to 350 Dobson units. Variation in atmospheric ozone at different latitudes [Data from P. S. Zurer, Chem. Eng. News, 24 May 1993 , p. 8.] By what percentage is the ultraviolet transmittance greater in winter than in summer?