(Solved): Thank you. In the book Flos, Fibonacci gave an approximation of the real number solution to the cubi ...

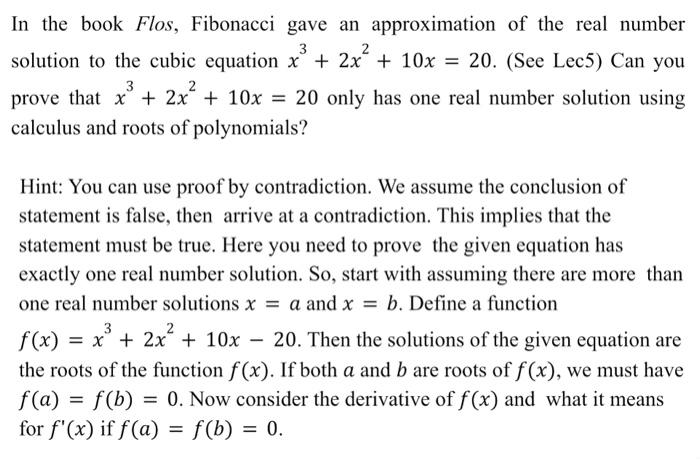
In the book Flos, Fibonacci gave an approximation of the real number solution to the cubic equation $x^3+2x^2+10x=20$. (See Lec5) Can you prove that $x^3+2x^2+10x=20$ only has one real number solution using calculus and roots of polynomials? Hint: You can use proof by contradiction. We assume the conclusion of statement is false, then arrive at a contradiction. This implies that the statement must be true. Here you need to prove the given equation has exactly one real number solution. So, start with assuming there are more than one real number solutions $x=a$ and $x=b$. Define a function $f(x)=x^3+2x^2+10x?20$. Then the solutions of the given equation are the roots of the function $f(x)$. If both $a$ and $b$ are roots of $f(x)$, we must have $f(a)=f(b)=0$. Now consider the derivative of $f(x)$ and what it means for $f^{?}(x)$ if $f(a)=f(b)=0$.