(Solved): Solve using the free body diagram. Problem 1: A pendulum consists of two \( 3.2-\mathrm{kg} \) sphe ...

Solve using the free body diagram.

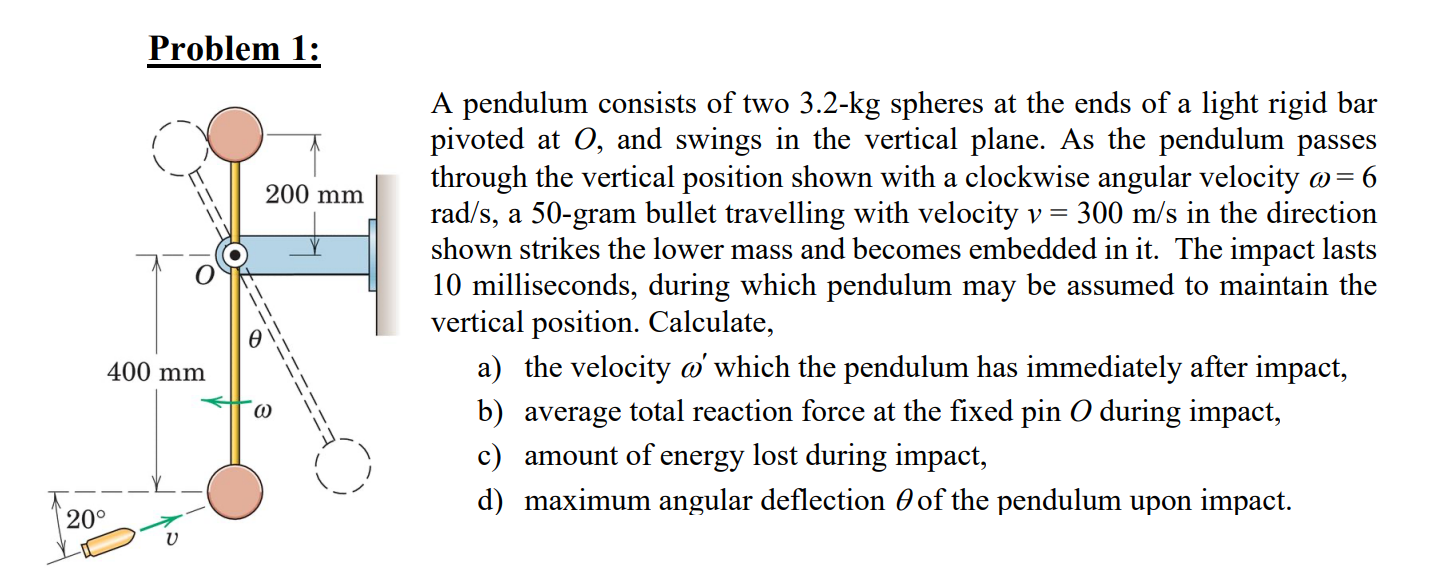
Problem 1: A pendulum consists of two \( 3.2-\mathrm{kg} \) spheres at the ends of a light rigid bar pivoted at \( O \), and swings in the vertical plane. As the pendulum passes through the vertical position shown with a clockwise angular velocity \( \omega=6 \) \( \mathrm{rad} / \mathrm{s} \), a 50 -gram bullet travelling with velocity \( v=300 \mathrm{~m} / \mathrm{s} \) in the direction shown strikes the lower mass and becomes embedded in it. The impact lasts 10 milliseconds, during which pendulum may be assumed to maintain the vertical position. Calculate, a) the velocity \( \omega^{\prime} \) which the pendulum has immediately after impact, b) average total reaction force at the fixed pin \( O \) during impact, c) amount of energy lost during impact, d) maximum angular deflection \( \theta \) of the pendulum upon impact.