(Solved): S2. Suppose that the mass in a spring-mass-dashpot system with m= 1, c= 2, and k= 8 is set in moti ...

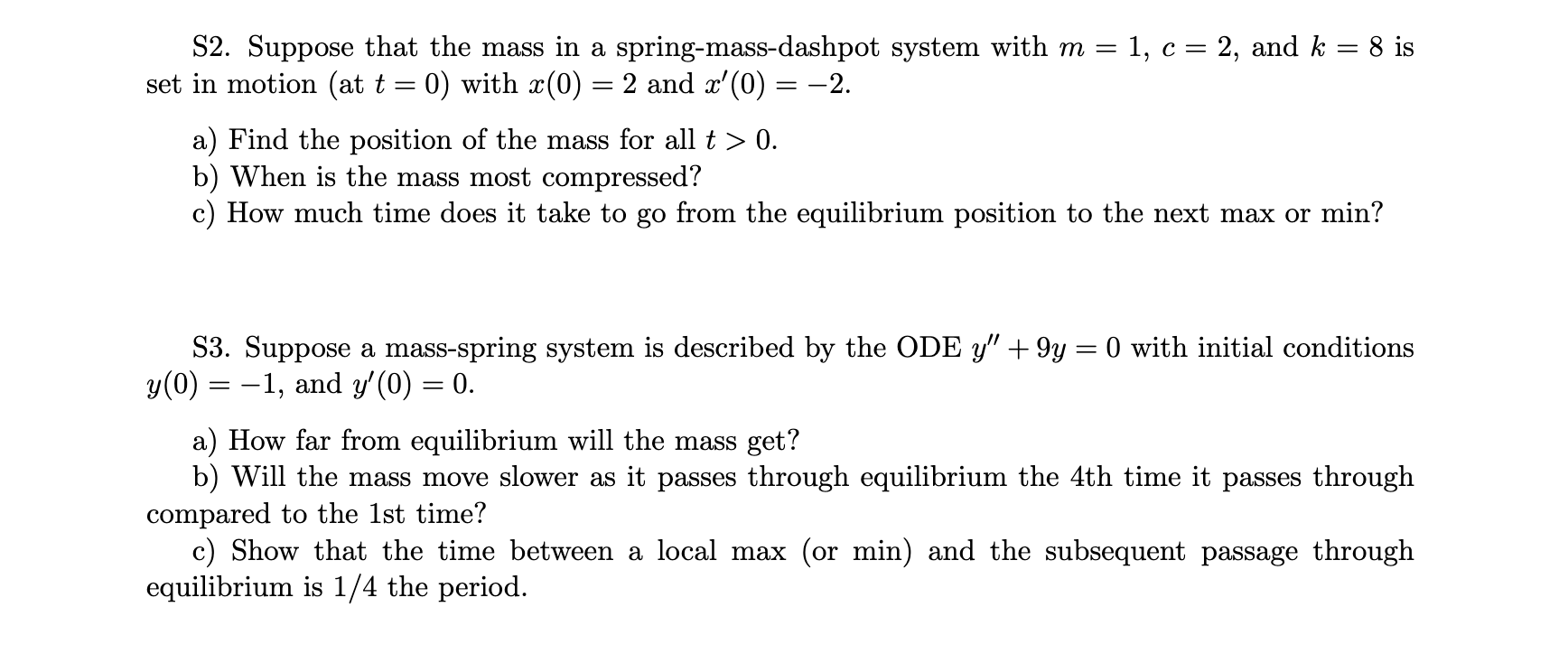
S2. Suppose that the mass in a spring-mass-dashpot system with m= 1, c= 2, and k= 8 is
set in motion (at t= 0) with x(0) = 2 and x0(0) = 2.
a) Find the position of the mass for all t>0.
b) When is the mass most compressed?
c) How much time does it take to go from the equilibrium position to the next max or min?

S3. Suppose a mass-spring system is described by the ODE y00+ 9 y= 0 with initial conditions
y(0) = 1, and y0(0) = 0.
a) How far from equilibrium will the mass get?
b) Will the mass move slower as it passes through equilibrium the 4th time it passes through
compared to the 1st time?
c) Show that the time between a local max (or min) and the subsequent passage through
equilibrium is 1/4 the period.

S2. Suppose that the mass in a spring-mass-dashpot system with \( m=1, c=2 \), and \( k=8 \) is set in motion (at \( t=0 \) ) with \( x(0)=2 \) and \( x^{\prime}(0)=-2 \). a) Find the position of the mass for all \( t>0 \). b) When is the mass most compressed? c) How much time does it take to go from the equilibrium position to the next max or min? S3. Suppose a mass-spring system is described by the ODE \( y^{\prime \prime}+9 y=0 \) with initial conditions \( y(0)=-1 \), and \( y^{\prime}(0)=0 \). a) How far from equilibrium will the mass get? b) Will the mass move slower as it passes through equilibrium the 4th time it passes through compared to the 1st time? c) Show that the time between a local max (or min) and the subsequent passage through equilibrium is \( 1 / 4 \) the period.