(Solved): QUESTION ONE 1. A toroid has a core of cross section \( 2500 \mathrm{~mm}^{2} \) in area and a mea ...

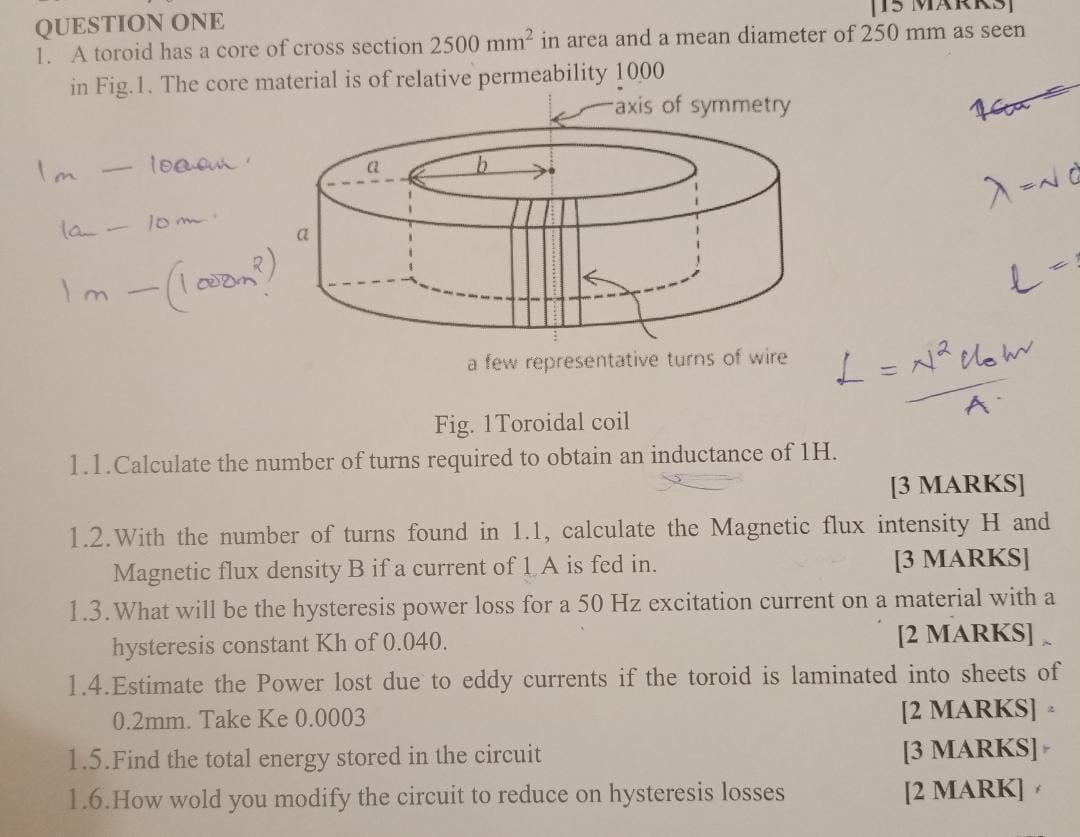
QUESTION ONE 1. A toroid has a core of cross section \( 2500 \mathrm{~mm}^{2} \) in area and a mean diameter of \( 250 \mathrm{~mm} \) as seen in Fig.1. The core material is of relative permeability 1000 In - 10quan. \( 10 m-10 m \). \( 1 m-\left(100 m^{2}\right) \) a few representative turns or wire \( \quad L=\frac{\text { Ho }^{2} \text { M }}{\text { H. }} \) Fig. 1Toroidal coil 1.1. Calculate the number of turns required to obtain an inductance of \( 1 \mathrm{H} \). [3 MARKS] 1.2. With the number of turns found in \( 1.1 \), calculate the Magnetic flux intensity \( \mathrm{H} \) and Magnetic flux density \( \mathrm{B} \) if a current of \( 1 \mathrm{~A} \) is fed in. [3 MARKS] 1.3. What will be the hysteresis power loss for a \( 50 \mathrm{~Hz} \) excitation current on a material with a hysteresis constant \( \mathrm{Kh} \) of \( 0.040 \). [2 MARKS] 1.4. Estimate the Power lost due to eddy currents if the toroid is laminated into sheets of \( 0.2 \mathrm{~mm} \). Take Ke \( 0.0003 \) [2 MARKS ] = 1.5. Find the total energy stored in the circuit [3 MARKS] 1.6. How wold you modify the circuit to reduce on hysteresis losses [2 MARK] ;