Home /
Expert Answers /
Computer Science /
question-2-the-cumulative-probability-distribution-function-of-a-continuous-random-variable-v-pa820
(Solved): Question 2. The cumulative probability distribution function of a continuous random variable \( V ...
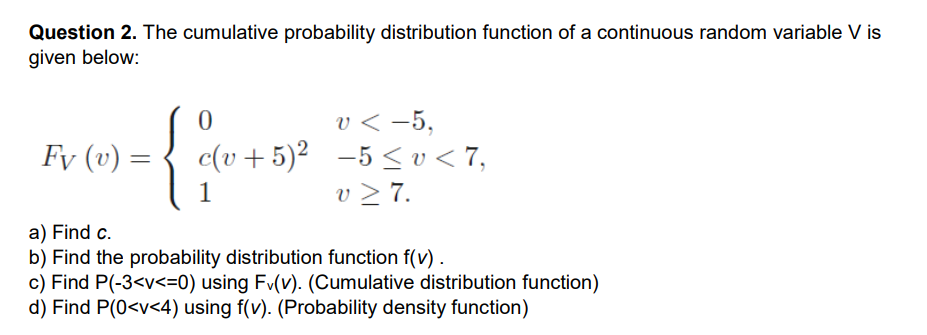
Question 2. The cumulative probability distribution function of a continuous random variable \( V \) is given below: \[ F_{V}(v)=\left\{\begin{array}{ll} 0 & v<-5 \\ c(v+5)^{2} & -5 \leq v<7 \\ 1 & v \geq 7 \end{array}\right. \] a) Find \( c \). b) Find the probability distribution function \( \mathrm{f}(v) \). c) Find \( \mathrm{P}(-3
Expert Answer
Question 1 c = 1/2 Question 2 The probability distribution function of a continuous random variable is given by: f(v) = F'(v) = 2(v+5) Therefore, the probability distribution function of the given random variable is: f(v) = 2(v+5) Question 3 P(-3