(Solved): QUESTION 2 Let the conditional \( N \), given \( Y \) has Poisson distributio ...

???????

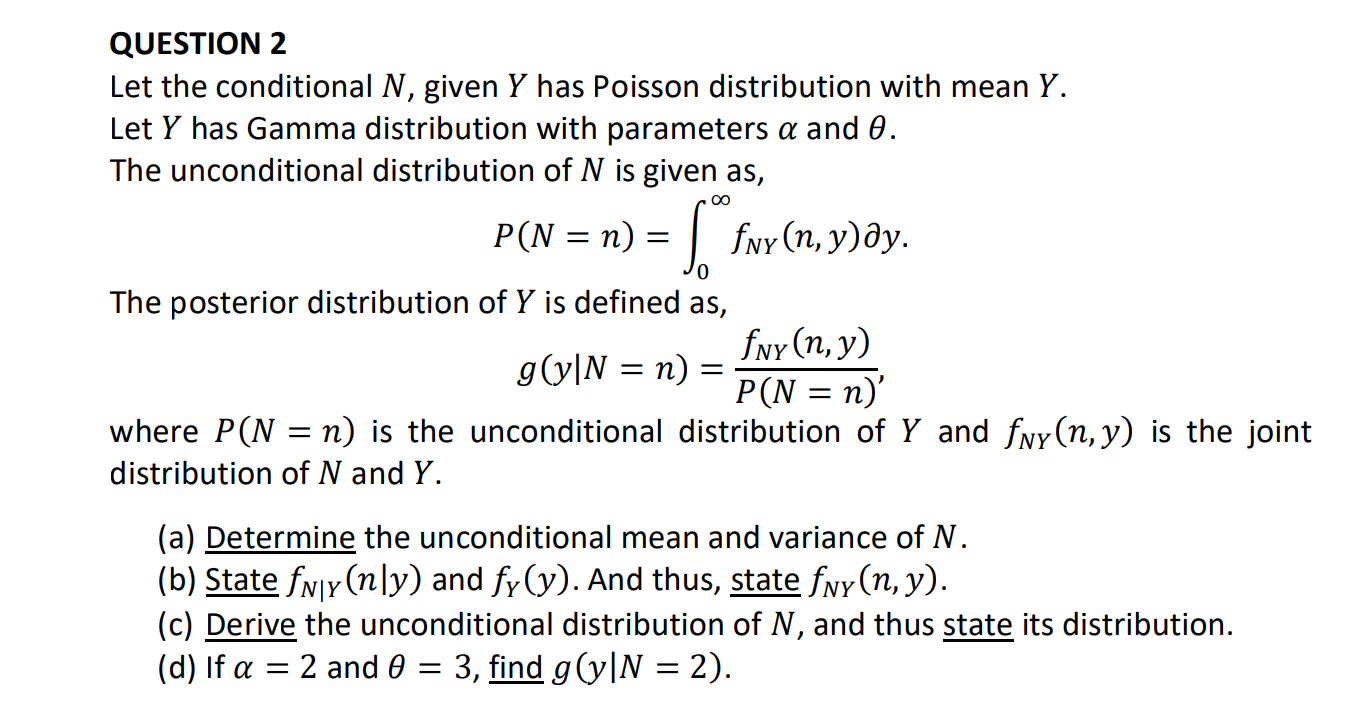
QUESTION 2 Let the conditional \( N \), given \( Y \) has Poisson distribution with mean \( Y \). Let \( Y \) has Gamma distribution with parameters \( \alpha \) and \( \theta \). The unconditional distribution of \( N \) is given as, \[ P(N=n)=\int_{0}^{\infty} f_{N Y}(n, y) \partial y . \] The posterior distribution of \( Y \) is defined as, \[ g(y \mid N=n)=\frac{f_{N Y}(n, y)}{P(N=n)}, \] where \( P(N=n) \) is the unconditional distribution of \( Y \) and \( f_{N Y}(n, y) \) is the joint distribution of \( N \) and \( Y \). (a) Determine the unconditional mean and variance of \( N \). (b) State \( f_{N \mid Y}(n \mid y) \) and \( f_{Y}(y) \). And thus, state \( f_{N Y}(n, y) \). (c) Derive the unconditional distribution of \( N \), and thus state its distribution. (d) If \( \alpha=2 \) and \( \theta=3 \), find \( g(y \mid N=2) \).