(Solved): Question 2: 25 points Consider fully developed laminar flow in a cylindrically intake to engine sho ...

Question 2: 25 points Consider fully developed laminar flow in a cylindrically intake to engine shown above. Also, let the pressure gradient, \( \frac{\partial p}{\partial z} \) be a constant and the outer radius \( \mathrm{R} \). (a) Reduce the Navier-Stokes equations given below in cylindrical coordinates to obtain a differential equation and the boundary conditions describing flow in the pipe. (10 points) B. ? (b) Perform the following tasks (i) Determine the velocity profile: (5 points) 2 (ii) Determine thestress at the wall and compare with the shear stress at the center line. 10 points) \[ \tau=\mu \frac{\partial v_{s}}{\partial \gamma} \] (iii) Determine the volumetric flow rate (10 points) \[ Q=\int \vec{V} \cdot \vec{A}=\int_{0}^{2} v_{z}(\gamma) \cdot 2 \pi \gamma \cdot 4 . \] (iv) What pressure gradient will result in no flow in the pipe? 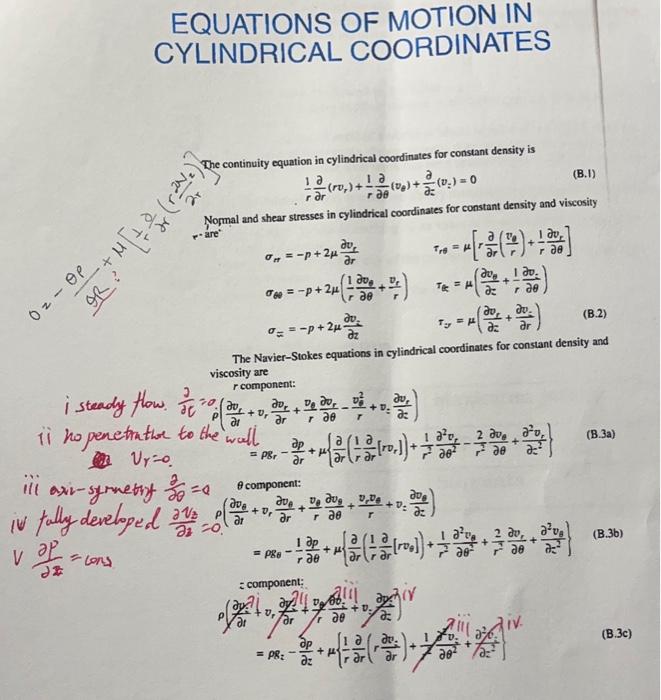(5 points) EQUATIONS OF MOTION IN CYLINDRICAL COORDINATES The continuity equation in cylindrical coordinates for constant density is \[ \frac{1}{r} \frac{\partial}{\partial r}\left(r v_{r}\right)+\frac{1}{r} \frac{\partial}{\partial \theta}\left(v_{e}\right)+\frac{\partial}{\partial z}\left(v_{z}\right)=0 \] Normal and shear stresses in cylindrical coordinates for constant density and viscosity \( e^{-} \)are' \[ \begin{array}{ll} \sigma_{r r}=-p+2 \mu \frac{\partial v_{r}}{\partial r} & \tau_{r \theta}=\mu\left[r \frac{\partial}{\partial r}\left(\frac{v_{\theta}}{r}\right)+\frac{1}{r} \frac{\partial v_{r}}{\partial \theta}\right] \\ \sigma_{\theta \theta}=-p+2 \mu\left(\frac{1}{r} \frac{\partial v_{\theta}}{\partial \theta}+\frac{v_{r}}{r}\right) & \tau_{k}=\mu\left(\frac{\partial v_{\theta}}{\partial z}+\frac{1}{r} \frac{\partial v_{z}}{\partial \theta}\right) \\ \sigma_{z=}=-p+2 \mu \frac{\partial v_{z}}{\partial z} & \tau_{r}=\mu\left(\frac{\partial v_{r}}{\partial z}+\frac{\partial v_{z}}{\partial r}\right) \end{array} \] The Navier-Stokes equations in cylindrical coordinates for constant density and viscosity are i steady flow. \( \frac{\partial}{\partial t}=0, \dot{\rho}\left(\frac{\partial v_{c}}{\partial t}+v_{r} \frac{\partial v_{r}}{\partial r}+\frac{v_{e}}{r} \frac{\partial v_{r}}{\partial \theta}-\frac{v_{o}^{2}}{r}+v_{z} \frac{\partial v_{r}}{\partial t}\right) \) ii ho penetint the to the wall \( =p_{r}-\frac{\partial p}{\partial r}+\mu\left\{\frac{\partial}{\partial r}\left(\frac{1}{r} \frac{\partial}{\partial r}\left[r r_{r}\right]\right)+\frac{1}{r^{2}} \frac{\partial^{2} v_{r}}{\partial \theta^{2}}-\frac{2}{r^{2}} \frac{\partial v_{\theta}}{\partial \theta}+\frac{\partial^{2} v_{r}}{\partial z^{2}}\right\} \) (B.3a) ii axi-syruetinf \( \frac{\partial}{\partial \theta}=0 \) ecomponent: fally developed \( \frac{\partial v_{z}}{\partial z}=\rho\left(\frac{\partial v_{\theta}}{\partial t}+v_{r} \frac{\partial v_{e}}{\partial r}+\frac{v_{e}}{r} \frac{\partial v_{\theta}}{\partial \theta}+\frac{v_{r} v_{\theta}}{r}+v_{z} \frac{\partial v_{\theta}}{\partial z}\right) \) \( \frac{\partial P}{\partial z}= \) cons \( =\rho g_{\theta}-\frac{1}{r} \frac{\partial p}{\partial \theta}+\mu\left\{\frac{\partial}{\partial r}\left(\frac{1}{r} \frac{\partial}{\partial r}\left[r v_{\theta}\right]\right)+\frac{1}{r^{2}} \frac{\partial^{2} v_{\theta}}{\partial \theta^{2}}+\frac{2}{r^{2}} \frac{\partial v_{r}}{\partial \theta}+\frac{\partial^{2} v_{\theta}}{\partial z^{2}}\right\} \) (B.3b)