(Solved): Question 1 Power Method converges to the largest eigenvalue while Inverse Power Method converges ...

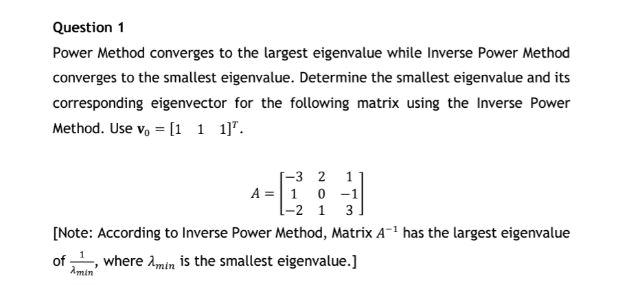
Question 1 Power Method converges to the largest eigenvalue while Inverse Power Method converges to the smallest eigenvalue. Determine the smallest eigenvalue and its corresponding eigenvector for the following matrix using the Inverse Power Method. Use $${\mathbf{v}}_0={\left[\begin{array}{lll}1& 1& 1\end{array}\right]}^T$$. $$A=\left[\begin{array}{ccc}?3& 2& 1\\ 1& 0& ?1\\ ?2& 1& 3\end{array}\right]$$ [Note: According to Inverse Power Method, Matrix $$A^{?1}$$ has the largest eigenvalue of $$\frac{1}{{?}_{\min }}$$, where $${?}_{\min }$$ is the smallest eigenvalue.] A ballistic missile launched by national defence security system with a velocity towards its target as: $$v(t)=2000\ln \left(\frac{14\times 10^4}{14\times 10^4?2100t}\right)?9.8t$$ where velocity, $$v$$ is given in $$\mathrm{m}/\mathrm{s}$$ and time, $$t$$ is given in seconds. (a) Use the forward, backward and central difference approximation of the first derivative of $$v(t)$$ to calculate the acceleration at $$t=16\mathrm{s}$$. Consider a step size of $$?t=2\mathrm{s}$$. (6 marks) (b) Compute the absolute relative true error in part (a) and give your comment on the results.