(Solved): quantum mechanics quantum 4.4. a. W ...

quantum mechanics

quantum

???????

???????

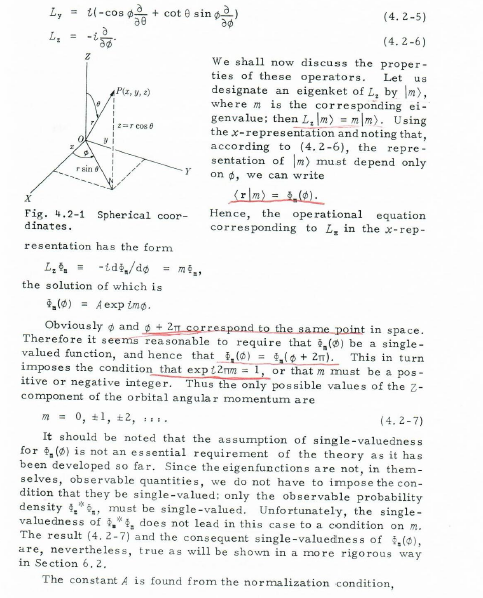
4.4. a. Write down the operator $$L_{+}$$in matrix fory for $$l=1$$ using the basis function $$Y_l$$ b. Find $$\left[L^2,L_{+}\right]$$ 4.2 Orbital Angular Momentum, The orbital angular momentum of a particle of momentum $$p$$ is $$L=r\times p$$. The corresponding quantal operator in the $$x$$-representation is $$L=?i?r\times ?$$. Choos $$?$$ ing thas the unit of angular momentum we shall write $$?\mathbf{L}=\mathbf{r}\times \mathrm{p}$$ so that the orbital angular momentum operator $$L=?ir\times ?$$ is dimensionless. This leads to a Z-component operator $$L=?i\left(x\frac{?}{?y}?y\frac{?}{?x}\right)$$ and to similar expressions for the other components of L. The commutation relations $$(3.4?15)$$ now become, independent of the representation used, $$\left[L_x,L_y\right]=t{L}_z\text{, etc., or }L\times L=tL$$ while the commutator $$\left[L^3,L_2\right]=0$$ obviously remains the same. For analyzing the motion under central forces it is more convenient to use spherical coordinates $$r,?,?(Fig.4.2?1)$$ instead of the rectangular coordinates $$x,y,z$$. The relation between the two sets is given by $$x=r\sin ?\cos ?,y=r\sin ?\sin ?,z=r\cos ?$$. Introducing the above change of variables into (4.2-2), we obtain $$L_x=i\left(\sin ?\frac{?}{??}+\cot ?\cos ?\frac{?}{??}\right)$$ $$\begin{array}{l}{L}_y=i\left(?\cos ?\frac{?}{??}+\cot ?\sin ?\frac{?}{??}\right)\\ L_z=?i\frac{?}{??}.\end{array}$$ We shall now discuss the properties of these operators. Let us designate an eigenket of $$L_2$$ by $$?\mathrm{m}?$$, where $$m$$ is the corresponding ei genvalue; then $$L_z?m?=m?m?$$. Using the $$x$$-representation and noting that, according to $$(4,2?6)$$, the representation of $$?m?$$ must depend only on $$?$$, we can write $$?\mathbf{r}?m?=3_n(?).$$ Fig. 4,2-1 Spherical coor- Hence, the operational equation dinates. corresponding to $$L_{\mathrm{z}}$$ in the $$x$$-representation has the form $$L_z{\hat{g}}_{\mathrm{n}}=??\mathrm{d}{?}_{\mathrm{m}}/\mathrm{d}?=m{\stackrel{?}{?}}_{\mathrm{n}},$$ the solution of which is $${?}_{\mathrm{a}}(?)=A\exp im?.$$ Obviously $$?$$ and $$?+2?$$ correspond to the same point in space. Therefore it seems reasonable to require that $${\stackrel{?}{q}}_n(?)$$ be a singlevalued function, and hence that $${?}_{?}(?)={?}_a(?+2?)$$. This in turn imposes the condition that $$\exp ?2?m=1$$, or that $$m$$ must be a pos itive or negative integer. Thus the only possible values of the Zcomponent of the orbital angular momentum are $$m=0,\pm 1,\pm 2,:\dots .$$ It should be noted that the assumption of single-valuedness for $${?}_{\mathrm{n}}(?)$$ is not an essential requirement of the theory as it has been developed so far. Since the eigenfunctions are not, in themselves, observable quantities, we do not have to impose the condition that they be single-valued; only the observable probability density $${\stackrel{?}{A}}_z{}^{?}{\stackrel{?}{?}}_n$$, must be single-valued. Unfortunately, the singlevaluedness of $${?}_m{}^{?}{?}_{\mathrm{z}}$$ does not lead in this case to a condition on $$\mathrm{m}$$. The result $$(4,2?7)$$ and the consequent single-valuedness of $${?}_2(?)$$, are, nevertheless, true as will be shown in a more rigorous way in Section 6,2. The constant $$A$$ is found from the normalization condition, $${?}_0^{2?}{\stackrel{?}{L}}_{\mathrm{i}}{?}_{\mathrm{z}}\mathrm{d}?={?}_0^{2?}{A}^{?}{\mathrm{e}}^{?1\mathrm{a}}{?}_A{e}^{1\mathrm{a}}{?}_{\mathrm{d}}\mathrm{d}=?A{?}^2{?}_0^{2?}\mathrm{d}?=2??A{?}^{\mathrm{a}}=1$$ giving $$A=(1/\sqrt{2?}){\mathrm{e}}^{18}$$. In general, however, we can assume that the phase $$?$$ is zero, in which case the normalized eigenfunctions for one of the components of the orbital angular momentum can be written simply as $${?}_{\mathrm{n}}(?)=(1/\sqrt{2?})\exp tm?$$. $$(4,2?8)$$ The result $$(4,2?7)$$ is significant because it shows that the component of orbital angular momentum along the $$Z$$ direction (or about any direction, since the Z-axis has been chosen arbitrarily) can take on only certain quantized values. This is equivalent to saying that a nonvanishing vector angular momentum can have only certain observable directions in space, all others being for bidden. This space quantization, as it is called, has a number of interesting physical implications, some of which will be examined later on. Having found the eigenvalues and functions corresponding to a component of the orbital angular momentum, we next investigate the eigenvalues of its magnitude, defined by $$L^3=L_x^2+L_y^2+L_{x^{?}}^2$$. We shall designate the eigenvalues of this operator by $$h_{\text{. . Keeping in }}$$ mind that $$L^3$$ and $$L_I$$ commute and therefore have a common set of kets $$??m?$$, , which we label by the eigenvalues to which they belong, we may write $$L^2??m?=???m?$$ as the eigenvalue equation for $$L^2$$. Evidently, $$?$$ must be a positive number. In addition we must have $$L_2??m?=m??m?$$. Using $$(4.2?4),(4.2?5)$$ and $$(4.2?6)$$, we can rewrite the operator $$L^2$$ in spherical coordinates as $$L^2=?\left\{\frac{1}{\sin ?}\frac{?}{??}\left(\sin ?\frac{?}{??}\right)+\frac{1}{{\sin }^3?}\frac{{?}^2}{?{?}^2}\right\}$$ or $$L^3=?\left\{\frac{1}{\sin ?}\frac{?}{??}\left(\sin ?\frac{?}{??}\right)=\frac{1}{{\sin }^3?}{L}_2^3\right\},$$ which clearly shows that $$L^a$$ and $$L_2$$ commute and thus that both operators have eigenfuctions with the same dependence on $$?$$. Of course the eigenfunctions of $${\mathrm{L}}^3$$ depend on $$?$$ and $$?$$ but not on $$r$$. Hence the common eigenfunctions of $$L^3$$ and $$L_z$$ are of the type $$?\mathrm{r}??m?=Y_{?=}(?,?)=P_{?\mathbf{s}}(?){\stackrel{?}{?}}_{\mathrm{s}}(?),$$ where $${\stackrel{?}{?}}_{\mathrm{a}}(?)$$ is given by $$(4,2?8)$$. Making this substitution in the eigenvalue equation [which now reads $$L^2{Y}_{?,s}(?,?)=?Y_{?,z}(?,?)]$$ and $$\sin ?\frac{d}{d?}\left(\sin ?\frac{d{P}_{?a}}{d?}\right)+\left(?{\sin }^2??m^2\right)P_{la}=0$$ If we substitute $$?=\cos ?$$, then this goes over into $$\left(1?{?}^2\right)P_{?n}?2?P_{?s}+\left[??\left(m^2/1?{?}^3\right)\right]P_{ln}=0,(4,2?11{)}^{\frac{d}{d}}$$ where we have designated by primes the derivatives with respect to $$?$$. To solve this equation we proceed as in the case of the harmonic oscillator, trying for $$P_{?a}(?)$$ a series development of the torm $$P_{hn}(?)={\left(1?{?}^2\right)}^{1=1/2}\left(a_0+a_{1?}+a_2{?}^2+?\right),(4,2?12)$$ and obtaining for the coefficients $$a_k$$, the recurrence equation, $$(?+1)(k+2)a_{k+2}=[(k+?m?+1)(k+?m?)??]a_k?(4,2?13)$$ Since it relates $$a_{\mathrm{k}}$$ and $$a_{\mathrm{k}+2}$$, the series for $$P_{?=}(?)$$ contains only alternate coefficients, either all even or all odd. An analysis of the convergence of the infinite series $$(4,2?12)$$ with coefficients related by $$(4.2?13)$$ shows that it diverges at the extremes of the interval of variation of $$?(?=\pm 1)$$. Therefore the series mustterminate and the solution $$P_{?}$$ must be a polynomial in $$?$$. Let us a 5 sume that $$a_2$$ is the last term, so that the polynomial is of degree $$h$$; then $$a_{k+2}=0$$ and $$?=(h+?m?)(h+?m?+1)$$. If we define $$\hat{i}=k+?m?=$$ positive integer $$k$$, then we find that the only possible values for the square of the orbital angular momentum a.re $$\left|L^2\right|=?=1(1+1),\{1=0,1,2,\dots =\text{ positive integer }\}.$$ For large values of $$l$$ one has approximately $$?\mathbf{L}??1$$. From this definition of 1 , we see that $$l??m?$$, a natural result, since the magnitude of the angular momentum, givenby $$I$$ cannot be sma ller than that of its components, given by m. Therefore, for a given $$l$$, $$m=0,\pm 1,\pm 2,\dots ,\pm (1?1),\pm 1,(4,2?15)$$ indicating that the angular momentum vector can have only $$2z+1$$ possible orientations. Since there are $$21+1$$ values of $$m$$ for each value of $$l$$, and therefore $$2l+1$$ different eigenfunctions, the angular momentum states corresponding to a given $$I$$ have a deg eneracy of order $$21+1$$. For reasons to be seen later (Section 4.11) spectroscopists have assigned letters to the different angular momentum states as follows: $$I=0,1,2,3,4,5,\dots$$ symbol; s, p, d, f, g, h, ... This is consistent with the condition $$\left(?m\left|L^2?L_z^2\right|?m\right)=??m^2?0$$. The degeneracy of the eigenvalues of $${\mathrm{L}}^2$$ with respect to $$m$$ has geometrical interpretation. It is obviously possible to change the $$\mathrm{Z}$$-component of a vector without changing its magnitude, by simply changing the orientation of the vector. However this degeneracy is, in a quantal sense, a direct consequence of the theorem stated in Problem 3.4-4. The fact that $${\mathrm{L}}^3$$ commutes with $$L_x,L_y$$ and $$L_z$$, but that these operators do not commute among themselves, requires that the eigenvalues of $$L^z$$ be degenerate. The solutions of $$(4,2?11)$$ with $$?=I(I+1)$$ are called associated Legendre functions, $$(1)$$ and are designated $$P_{1n}(4)$$. They can be expressed in the closed form (see Appendix C. 2) $$P_{1z}(?)={\left(1?{?}^2\right)}^a/a{\mathrm{d}}^{?}{P}_1(?)/\mathrm{d}{?}^a(0?m?1)$$ $$(4,2?16)$$ where $$P_1(?)=\frac{1}{2^{1}1!}\frac{d^1}{d?}{\left({?}^3?1\right)}^1$$ is called a Legendre polynomial of degree 1 . It contains only terms with powers $$1,1?2,1?4,\dots ,1$$ or 0 . Combining $$(4,2?16)$$ and $$(4,2?17)$$ and remembering that $$?=$$ $$\cos ?$$, we can write the alternative expression $$P_{1s}(\cos ?)=\frac{(?{)}^1}{2^{1}1!}{\sin }^2?\frac{d^{1+?}\left({\sin }^{31}?\right)}{d(\cos ?{)}^{1+z}}(0?m?1)\text{. }$$ This form may now be used to extend the definition of $$P_1$$ to the region $$?1?m?0$$. Since $$(4,2?11$$ ) depends only on $$m^2$$, its regular $$($$ at $$?=\pm 1$$ ) solutions, $$P_{1n}$$ and $$P_{t=n}$$, must be linearly related, i. e., $$P_{1a}=C{P}_{1a}$$. The constant $$C$$ may be evaluated directly by equating the highest powers of $$P_{1s}$$ and $$P_{1??}$$ as defined by $$(4,2?18)$$. The properly normalized solutions of $$(4,2?11)$$ are designated by $${?}_{1n}$$, and are called normalized associated Legendre-funetions. They may be written as $$P_1(u)=N_{1a}{P}_{1u}(?)$$ where $$N_1$$ is the norma lization constant, $$N_1=\sqrt{\frac{21+1}{2}\frac{(1?m)!}{(1+m)!}}$$ obtained by requiring $${?}_{?1}^{+1}{\left[{?}_{1n}(?)\right]}^3\mathrm{d}?=1\text{. }$$ $$(4.2?19)$$ Note that since the polynomial appearing in $$(4.2=16)$$ is of degree $$1?m$$ and contains only alternate powers of $$?$$, it follows that $${?}_{1a}(??)=(?{)}^{1??}{?}_{1?}(?).$$ It may be verified from their definitions that the normalized functions satisfy the condition (see also Problem 4.2-7) $${?}_{1,?\pm }(?)=(?{)}^n{?}_1(?).$$ Whiththese results we may write the common eigenfunctions of $${\mathrm{L}}^2$$ and $$L_z$$ as $$Y_{1a}(?,?)=(1/\sqrt{2?}){?}_{1\pm }(\cos ?)\exp im?.$$ The functions defined by Eq. $$(4,2?23)$$ are called the normalized spherical harmonics. The spherical harmonics for $$l=0,1$$ and 2 are given in Table $$4,2?1$$. $$\begin{array}{c}\text{ Table 4.2-1 Angular Functions Corresponding }\\ \text{ to }{\mathbf{L}}^2\text{ and }{L}_v\end{array}$$ Some properties of these functions are: $$\begin{array}{l}{L}^2{Y}_1,(?,?)=I(1+1)Y_{1?}(?,?)\\ (4.2?24)\\ L_z{Y}_{1n}(?,?)=m{Y}_{1z}(?,?)\\ (4.2?25)\\ Y_{1a}^{?}(?,?)=(?{)}^{\pm }{F}_{1?s}(?,?)\\ (4,2?26)\\ {?}_{1\pm }(???,?+?)?(?{)}^2{Y}_{1:}(?,?)\\ (4.2?27)\\ Y_{10}(?,?)=(2l+1/4?{)}^{\frac{1}{2}}{P}_2(\cos ?)\\ (4,2?28)\\ Y_{L?}(0,?)=?2I+1/4??\text{ ?? }{?}_{\mathrm{I}}\\ (4,2?29)\\ ?Y_{1s}(?,?)Y_1{}^{1}1(?,?)s\mathrm{in}?d?d?={?}_{11^6}{}^{?}=1.(4,2?30)\end{array}$$ The result $$(4,2?27)$$ is obtained by combining $$(4.2?21)$$ with the relation $$\exp tm(?+?))=(?{)}^{?}\exp tm?$$. Equation $$(4.2?30)$$ is the nor - The parity operation $$P$$, that is the replacement of $$r$$ by $$?r$$, is equivalent in polar coordinates to replacing $$r,?,?$$ by $$r,?=?$$, $$?+?$$, Hence, making use of $$(4.2?27)$$ $$P{Y}_{La}(?,?)=Y_{1?}(???,?+?)=(?{)}^1{Y}_I(?,?).$$ The result shows that the angular momentum states have a welldefined parity determined solely by 1 . The states are even or odd according to whether $$l$$ is even or odd completely independent of $$m$$. That the angular momentum states have a well-defined parity is not surprising, Observe that the $$P$$ operation which changes $$r$$ into $$?r$$, also changes $$p$$ into $$?p$$ or $$?$$ into $$??$$, But this means that $$AL=r\times P$$ is invariant to the $$P$$ ope ration, $$i.e.,PL=$$ $$=$$ LP or $$[L,P]=0$$. Vectors with this property are called axial vectors, Hence, the eigenfunctions of $$L^3$$ are also eigenfunctions of $$\mathrm{P}$$ and have well-defined parity For future reference we list here some additional useful relations: $$\begin{array}{l}\begin{array}{l}{?}_{?1}^{+1}{P}_1(?)P_1(?)\mathrm{d}?=2{?}_{11}1/(21+1)\\ {?}_{?1}^{+1}{?}_1(?){?}_1{1}_1(?)d?={?}_{111}\end{array}\\ \sin ?{\mathrm{e}}^1{?}_{Y_{1n}}=?{\left[\frac{(1+m+1)(1+m+2)}{(21+1)(21+3)}\right]}^{\frac{1}{2}}{Y}_{1+1,\mathrm{o}+1}+\\ +{\left[\frac{(1?m)(1?m?1)}{(21+1)(21?1)}\right]}^{\frac{1}{8}}{Y}_{1?1,n+1}\\ \sin {\mathrm{se}}^{?1?}{Y}_{1s}={\left[\frac{(1?m+1)(1?m+2)}{(21+1)(2l+3)}\right]}^{\frac{1}{2}}{Y}_{1+1,=?1}\\ ?{\left[\frac{(1+m)(2+m?1)}{(21+1)(2)?1)}\right]}^{\frac{1}{2}}{Y}_{1?19m?1}\\ \cos ?Y_{Ls}=\left[\frac{(1+m+1)(1?m+1)}{(21+1)(21+3)}\right]Y_{L+1,{\mathbf{a}}^2}+\left[\frac{(1+m)(1?m)}{(21+1)(21?1)}\right]Y_{1?1},\mathrm{m}\\ (4,2?35)\\ \text{ Defining the operators }\\ L_{+}=L_x+i{L}_y=e^{12t}(z/??+t\cot ??/??)(4.2?36)\\ L_{?}=L_x?i{L}_{\mathrm{y}}??e^{?1?(}?/???i\cot ?//??),\end{array}$$ one can verify that $$L_{\pm }{Y}_{1\mathrm{a}}=[(1?m)(2\pm m+1){]}^{\frac{2}{2}}{V}_{1{}_{\pm 1}}$$ so that the operators $$L_{\pm }$$carry one from the function $$Y_{1a}$$ to a function proportional to $$F_1\pm 1^{?}$$. These operators and relations arespecial cases of more general ones to be discussed in Chapter VI. From $$(4,2?26)$$, we see that $${?Y_{\mathrm{Ln}}?}^8={?Y_{1=\mathrm{e}}?}^{\text{andicating that the }}$$ spatial distribution for a given $$l$$ is the same for either $$+\mathrm{m}$$ or $$?m$$. This is due to the fact that only $$m^2$$ appears in Eq. $$(4.2?11)$$, or equiva lently because $$L^2$$ depends only on $$L_{x^2}$$. Physically this result arises from the impossibility of distinguishing between the directions $$+Z$$ and $$?Z$$ in an isolated system. It follows in such cases that we can equally well use any linea $$r$$ combination $$a{Y}_{1s}+b{Y}_{1?z}$$ as an eigenfunction of $$L^2$$, the most convenient being the real functions $$\begin{array}{l}{Y}_{100}=Y_{10,}\\ {?}_{11=1}(+)=(1/\sqrt{2})\left[Y_{1n}+(?{)}^2{Y}_{1?n}\right\}=(1/\sqrt{?}){?}_{10}(\cos ?)\cos m{?}_2\\ {?}_{1?=1}(?)=(1/t\sqrt{2}]\left[{?}_1=?(?{)}^n{V}_{2?s}\right]=(4,2?39)\\ \text{ Selected in this }\end{array}$$ not of $$L_2$$ in this way the $${?}_1?t$$ are eigenfunctions of $$L^2$$ and $$L_z^8$$ but ject to some of hotd be noted, however, that if the system is subject to 8 ome external action that distinguishes between $$+Z$$ and $$?Z$$, such as a magnetic or electric field, then we, in general, cannot use such eigenfuentions as $$(4.2?39)$$ and must instead return to those in $$(4.2?23)$$.