Home /
Expert Answers /
Statistics and Probability /
q3-consider-the-class-means-and-covariance-matrices-for-classes-q-1-and-c-2-mu-1-1-3-m-pa864
(Solved): Q3. Consider the class means and covariance matrices for classes q_(1) and c_(2) : \mu _(1)=(1,3),\m ...
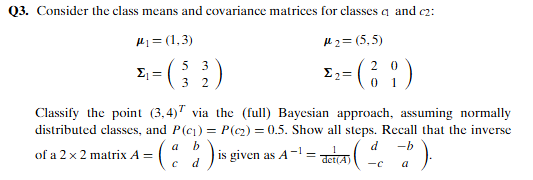
Q3. Consider the class means and covariance matrices for classes q_(1) and c_(2) :
\mu _(1)=(1,3),\mu _(2)=(5,5)
\Sigma _(1)=([5,3
3,2]),\Sigma _(2)=([2,0
0,1])
Classify the point (3,4)^(T) via the (full) Bayesian approach, assuming normally
distributed classes, and P(c_(1))=P(c_(2))=0.5. Show all steps. Recall that the inverse
of a 2\times 2 matrix A=([a,b],[c,d]) is given as A^(-1)=(1)/(det(A))([d,-b],[-c,a]).