(Solved): q10 This rule is used in two ways to convert biconditional statements. First, the material equivale ...

q10

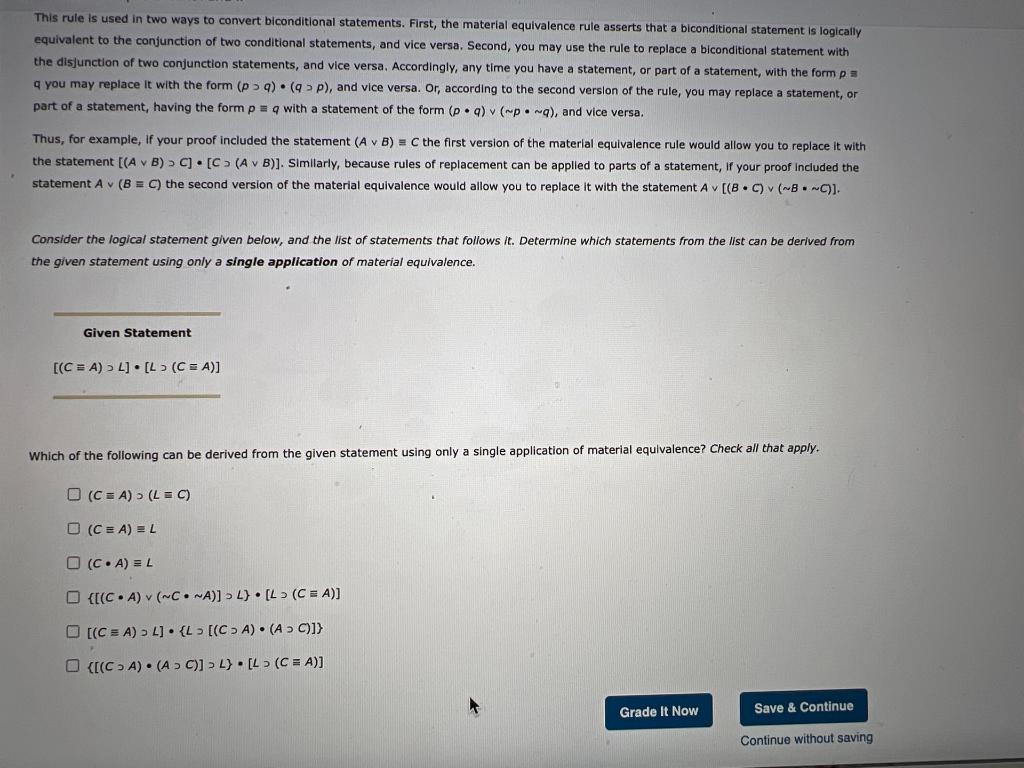
This rule is used in two ways to convert biconditional statements. First, the material equivalence rule asserts that a biconditional statement is logically equivalent to the conjunction of two conditional statements, and vice versa, Second, you may use the rule to replace a biconditional statement with the disjunction of two conjunction statements, and vice versa. Accordingly, any time you have a statement, or part of a statement, with the form \( p \); \( q \) you may replace it with the form \( (p>q) \cdot(q \supset p) \), and vice versa. Or, according to the second version of the rule, you may replace a statement, or part of a statement, having the form \( p \equiv q \) with a statement of the form \( (p \cdot q) \vee(\sim p \bullet \sim q) \), and vice versa. Thus, for example, If your proof included the statement \( (A \vee B) \equiv C \) the first version of the material equivalence rule would allow you to replace it with the statement \( [(A \vee B) \supset C] \cdot[C \supset(A \vee B)] \). Similarly, because rules of replacement can be applled to parts of a statement, if your proof included the statement \( A \vee(B \equiv C) \) the second version of the material equivalence would allow you to replace it with the statement \( A \vee[(B \cdot C) \vee(\sim B \) * \( \sim C)] \). Consider the logical statement given below, and the list of statements that follows it. Determine which statements from the list can be derived from the given statement using only a single application of material equivalence. Given Statement \[ [(C \equiv A)>L] \cdot[L>(C \equiv A)] \] Which of the following can be derived from the given statement using only a single application of material equivalence? Check all that apply. \[ (C \equiv A)>(L \equiv C) \] \( (C \equiv A) \equiv L \) \( (C \cdot A) \equiv L \) \( \{[(C \cdot A) \vee(\sim C \cdot \sim A)] \supset L\} \cdot[L \supset(C \equiv A)] \) \[ \begin{array}{l} {[(C \equiv A)>L] \cdot\{L \supset[(C, A) \cdot(A \supset C)]\}} \\ \{[(C, A) \cdot(A, C)]>L\} \cdot[L \supset(C \equiv A)] \end{array} \]