(Solved): Problem 7-18. Two-Dimensional Creeping Flow (a) Consider the 2D flow of a ribbon of fluid confined ...

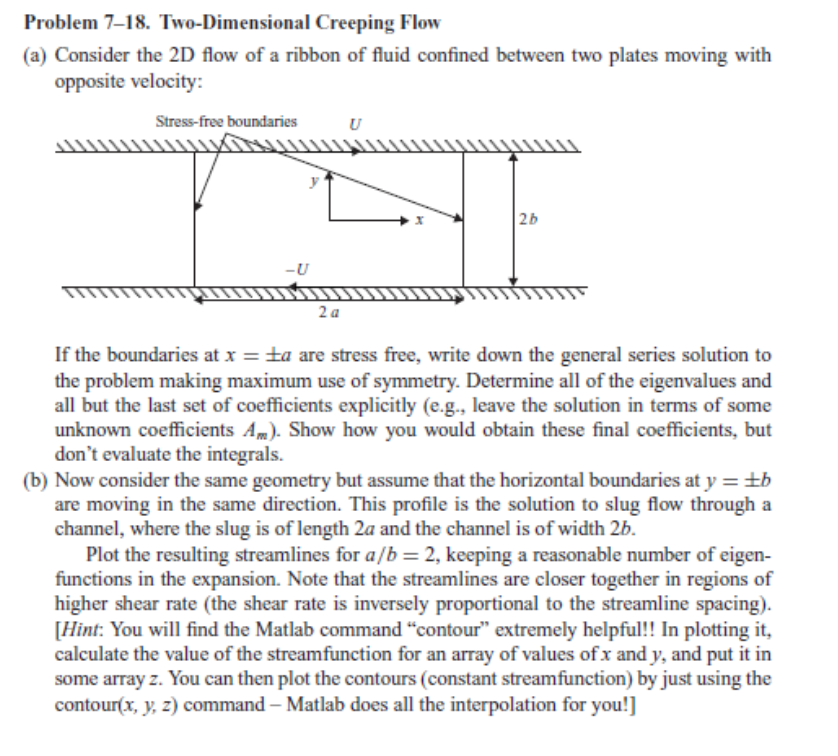
Problem 7-18. Two-Dimensional Creeping Flow (a) Consider the 2D flow of a ribbon of fluid confined between two plates moving with opposite velocity: Stress-free boundaries U 2b 2 a If the boundaries at x = ta are stress free, write down the general series solution to the problem making maximum use of symmetry. Determine all of the eigenvalues and all but the last set of coefficients explicitly (e.g., leave the solution in terms of some unknown coefficients Am). Show how you would obtain these final coefficients, but don't evaluate the integrals. (b) Now consider the same geometry but assume that the horizontal boundaries at y=+b are moving in the same direction. This profile is the solution to slug flow through a channel, where the slug is of length 2a and the channel is of width 2b. Plot the resulting streamlines for a/b = 2, keeping a reasonable number of eigen- functions in the expansion. Note that the streamlines are closer together in regions of higher shear rate (the shear rate is inversely proportional to the streamline spacing). [Hint: You will find the Matlab command "contour" extremely helpful!! In plotting it, calculate the value of the streamfunction for an array of values of x and y, and put it in some array z. You can then plot the contours (constant stream function) by just using the contour(x, y, z) command - Matlab does all the interpolation for you!] ?? y -U