(Solved): Problem 4: Modelling Physical Systems Using ODEs Consider the system shown below In this system, th ...

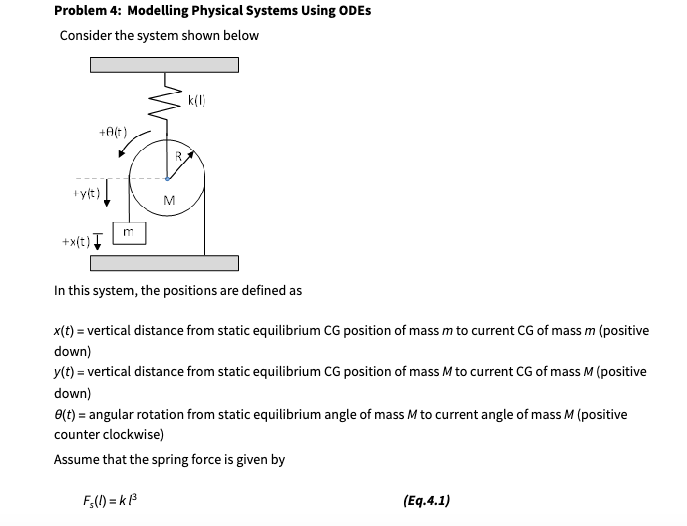
Problem 4: Modelling Physical Systems Using ODEs Consider the system shown below In this system, the positions are defined as $x(t)=$ vertical distance from static equilibrium CG position of mass $m$ to current CG of mass $m$ (positive down) $y(t)=$ vertical distance from static equilibrium CG position of mass $M$ to current CG of mass $M$ (positive down) $?(t)=$ angular rotation from static equilibrium angle of mass $M$ to current angle of mass $M$ (positive counter clockwise) Assume that the spring force is given by $F_5(l)=k{l}^3$ where $l=$ spring length - relaxed spring length Part a. Draw a picture of the system unloaded (with no masses) and next to it draw a picture of the system loaded but at rest. Draw a free body diagram of the pulley at rest to find $?$, the deflection of the spring from the unloaded position to the loaded equilibrium position. Part b. Derive the equation of motion for the system. Assume that $J=\frac{1}{2}M{R}^2$ (pulley is a thin disc) Hint 1: Determine how $x(t)$ and $?(t)$ are related to $y(t)$ and write all equations in terms of $y(t)$ Hint 2: After substituting in $J=\frac{1}{2}M{R}^2$, all the $R$ terms in your equation should cancel. Part c. Explain why the system is non-linear if the spring force is given by Eq.4.1. Part d. Numerically solve the non-linear system derived in part a using Simulink. Simulate the system with initial conditions of $y(0)=2,\stackrel{?}{y}(0)=?1$ and constants of $\begin{array}{l}M=1\\ m=1/2\\ k=3\\ g=9.81\end{array}$ Part e. Explain why the system becomes linear if we instead approximate the spring force as $F_s(l)=kl$ (Eq.4.2) Part $f$. Solve the linear system from part e analytically for $y(t)$ with the same initial conditions as part $d$. Part g. Numerically solve the linear system derived in part e using Simulink with the same initial conditions as part $\mathrm{d}$. Part h. Compare and contrast the linear and non-linear models. Comment on their complexity and fidelity.