(Solved): Problem 4. (Entanglement Swapping) In the Quantum Teleportation protocol, Alice and Bob start with ...

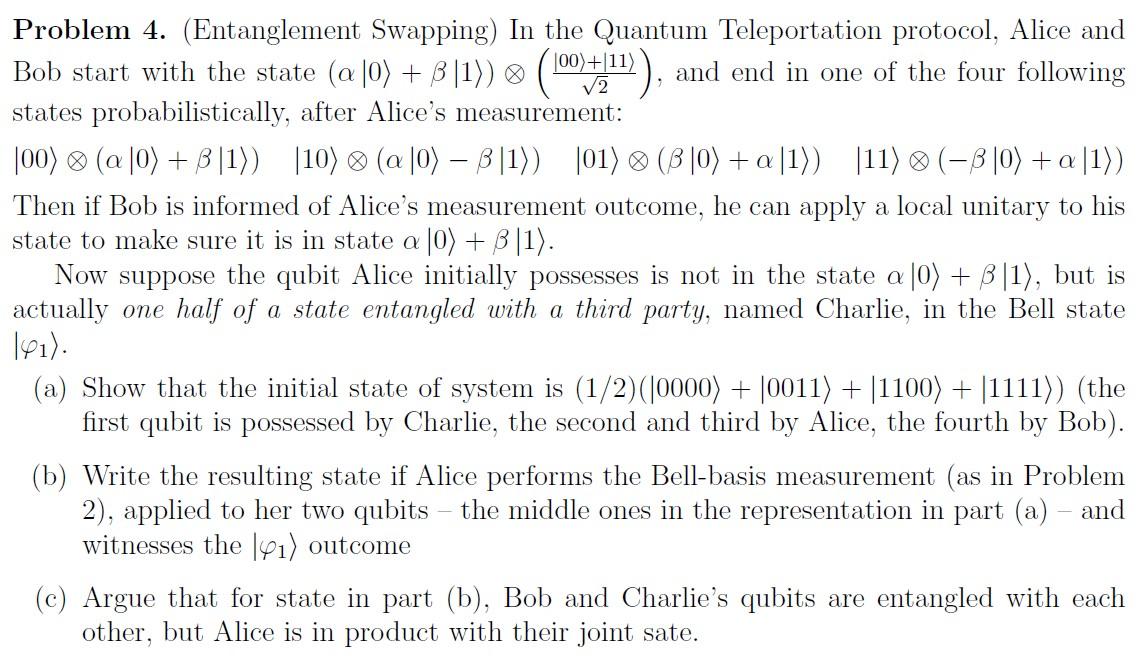
Problem 4. (Entanglement Swapping) In the Quantum Teleportation protocol, Alice and Bob start with the state $(??0?+??1?)?\left(\frac{?00?+?11?}{\sqrt{2}}\right)$, and end in one of the four following states probabilistically, after Alice's measurement: $?00??(??0?+??1?)?10??(??0????1?)?01??(??0?+??1?)?11??(???0?+??1?)$ Then if Bob is informed of Alice's measurement outcome, he can apply a local unitary to his state to make sure it is in state $??0?+??1?$. Now suppose the qubit Alice initially possesses is not in the state $??0?+??1?$, but is actually one half of a state entangled with a third party, named Charlie, in the Bell state $?{?}_1?$ (a) Show that the initial state of system is $(1/2)(?0000?+?0011?+?1100?+?1111?)$ (the first qubit is possessed by Charlie, the second and third by Alice, the fourth by Bob). (b) Write the resulting state if Alice performs the Bell-basis measurement (as in Problem 2), applied to her two qubits - the middle ones in the representation in part (a) - and witnesses the $?{?}_1?$ outcome (c) Argue that for state in part (b), Bob and Charlie's qubits are entangled with each other, but Alice is in product with their joint sate.