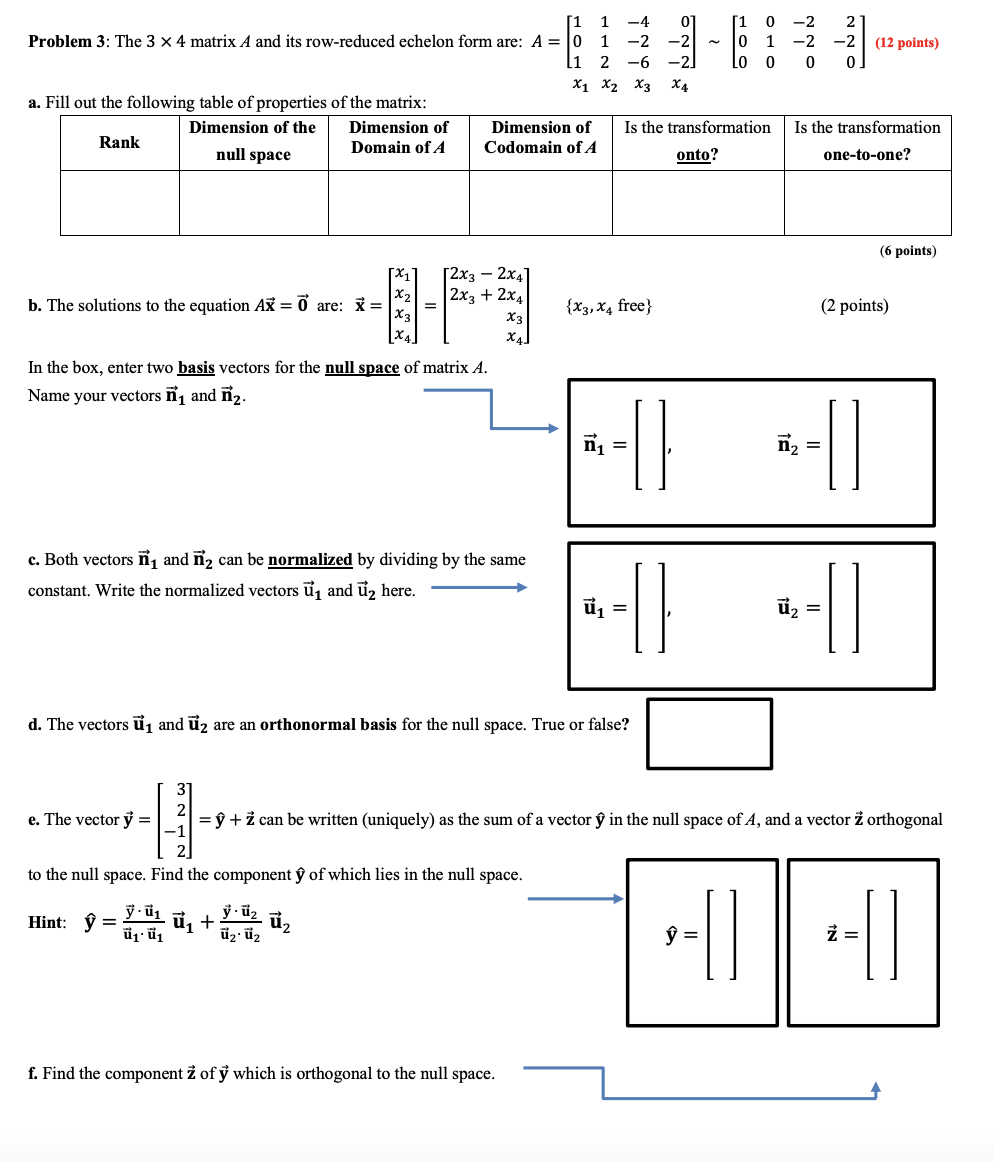
(Solved): Problem 3: The \( 3 \times 4 \) matrix \( A \) and its row-reduced echelon form are: \( A=\left[\b ...

Problem 3: The \( 3 \times 4 \) matrix \( A \) and its row-reduced echelon form are: \( A=\left[\begin{array}{rrrr}1 & 1 & -4 & 0 \\ 0 & 1 & -2 & -2 \\ 1 & 2 & -6 & -2\end{array}\right] \sim\left[\begin{array}{rrrr}1 & 0 & -2 & 2 \\ 0 & 1 & -2 & -2 \\ 0 & 0 & 0 & 0\end{array}\right] \) (12 points) a. Fill out the following table of properties of the matrix: b. The solutions to the equation \( A \overrightarrow{\mathbf{x}}=\overrightarrow{\mathbf{0}} \) are: \( \overrightarrow{\mathbf{x}}=\left[\begin{array}{l}x_{1} \\ x_{2} \\ x_{3} \\ x_{4}\end{array}\right]=\left[\begin{array}{r}2 x_{3}-2 x_{4} \\ 2 x_{3}+2 x_{4} \\ x_{3} \\ x_{4}\end{array}\right] \quad\left\{x_{3}, x_{4}\right. \) free \( \} \) (6 points) In the box, enter two basis vectors for the null space of matrix \( A \). Name your vectors \( \overrightarrow{\mathbf{n}}_{\mathbf{1}} \) and \( \overrightarrow{\mathbf{n}}_{\mathbf{2}} \). ( 2 points) c. Both vectors \( \overrightarrow{\mathbf{n}}_{\mathbf{1}} \) and \( \overrightarrow{\mathbf{n}}_{\mathbf{2}} \) can be normalized by dividing by the same constant. Write the normalized vectors \( \vec{u}_{1} \) and \( \overrightarrow{\mathrm{u}}_{2} \) here. \( \overrightarrow{\mathbf{u}}_{1}=[], \quad \overrightarrow{\mathbf{u}}_{2}=[] \) d. The vectors \( \overrightarrow{\mathbf{u}}_{\mathbf{1}} \) and \( \overrightarrow{\mathbf{u}}_{\mathbf{2}} \) are an orthonormal basis for the null space. True or false? \[ \overrightarrow{\mathbf{n}}_{2}=[] \] e. The vector \( \overrightarrow{\mathbf{y}}=\left[\begin{array}{r}3 \\ 2 \\ -1 \\ 2\end{array}\right]=\hat{\mathbf{y}}+\overrightarrow{\mathbf{z}} \) can be written (uniquely) as the sum of a vector \( \hat{\mathbf{y}} \) in the null space of \( A \), and a vector \( \overrightarrow{\mathbf{z}} \) orthogonal to the null space. Find the component \( \hat{y} \) of which lies in the null spac Hint: \( \quad \hat{\mathbf{y}}=\frac{\overrightarrow{\mathrm{y}} \cdot \overrightarrow{\mathrm{u}}_{1}}{\overrightarrow{\mathrm{u}}_{1} \cdot \overrightarrow{\mathrm{u}}_{1}} \overrightarrow{\mathbf{u}}_{1}+\frac{\overrightarrow{\mathrm{y}} \cdot \overrightarrow{\mathrm{u}}_{2}}{\overrightarrow{\mathbf{u}}_{2} \cdot \overrightarrow{\mathrm{u}}_{2}} \overrightarrow{\mathbf{u}}_{2} \) f. Find the component \( \overrightarrow{\mathbf{z}} \) of \( \overrightarrow{\mathbf{y}} \) which is orthogonal to the null space.